Basic concepts on problems on speed time distance, train running and boats in rivers

Submitted by Atanu Chaudhuri on Tue, 12/07/2016 - 11:03

Speed time distance concepts govern the running of trains and plying of boats in rivers as well

Speed time distance train running boats in rivers

Traveling at same speed we would need twice the time we took last time, if we have to cover twice the distance we traveled last time. This follows from our common sense. The relationship between speed, time and distance is one of the most basic physical laws that affects our real life experiences extensively.

Trains run from station to station at various speeds; boats ply in the river but their actual speeds are reduced by the river stream speed if the boat moves upstream...the applications of the relationship between speed time and distance form an important part of Arithmetic.

Speed, time and distance - basic concepts

Speed is the distance traversed per unit time by an object moving in the same direction. The relation between Speed, Distance and Time is,

\[ \text{Speed} = \frac{\text{Distance}}{\text{Time}} \]

If Time is held constant, Speed is directly proportional to Distance. If Speed is more, the Distance traversed will be more during the same period.

\[ \text{If Time is constant,}\quad \text{Speed} \propto \text{Distance} \]

If Distance is held constant, Speed is inversely proportional to Time. It means, to cover the same distance it would take less time if the speed is increased.

\[ \text{If Distance is constant,} \quad \text{Speed} \propto \frac{1}{\text{Time}} \]

If Speed is held constant, Distance covered is directly proportional to Time. In other words, at constant speed, distance traversed will be more in more time.

\[ \text{If Speed is constant,} \quad \text{Distance} \propto \text{Time} \]

Relative speed and catching up:

If two objects move in parallel paths in same direction with speeds $S_1$ and $S_2$, with the first speed higher, the relative speed between the two will be $S_1 - S_2$.

If object 2 was ahead of object 1 by a distance $d$, the first object will catch up with the second in time,

\[ T=\frac{d}{S_1 - S_2} \]

Effectively object 2 can be imagined to be stationary at a distance $d$ from object 1, and object 1 approaches object 2 at the relative speed $S_1 - S_2$ catching it up in time $T$.

As in time $T$ object 1 moves a distance of $T\times{S_1}$, this will be distance from the starting point of object 1 at which the two objects will meet.

In reality, during this time $T$, object 2 will also move with its speed $S_2$ and will meet object 1 at a point distant $T\times{S_2}$ from its own starting point.

Problem examples

Problem 1:

A person covers one-third of his journey at 40 km per hour, one half of the journey at 30 km per hour and rest at 20 km per hour. What was his average speed for the whole journey?

Solution 1:

Rest of the journey was $\displaystyle\left(1 - \frac{1}{2} - \frac{1}{3}\right)= \frac{1}{6}$th of the journey that was covered at speed 20 km per hour.

So, if Distance was $D$ kms, total time taken in hours was,

$\text{Time}=\displaystyle\frac{D}{3\times{40}} + \displaystyle\frac{D}{2\times{30}} + \displaystyle\frac{D}{6\times{20}}$

$\hspace{12mm}=D\left(\displaystyle\frac{1}{120} + \displaystyle\frac{1}{60} + \displaystyle\frac{1}{120}\right)$

$\hspace{12mm}=\displaystyle\frac{D}{30}$.

So, the average speed was 30 kms per hour.

Problem 2:

A bee flies from its hive to a flower in a straight path at a speed of 30 km per hour and returns immediately to its hive following the same path at a liesurely speed of 10 km per hour. What was its average speed?

Solution 2:

If the distance was $D$, the total time taken to cover the total distance $2D$ in hours was,

$ \text{Time } = \displaystyle\frac{D}{30} + \displaystyle\frac{D}{10}$

$\hspace{13mm}=\displaystyle\frac{4D}{30}=\frac{2D}{\displaystyle\frac{30}{2}}=\frac{2D}{15}$.

So the average speed of the bee was 15 km per hour.

Problem 3 - Catching up:

Raman runs at a speed of 24 kms/hr and Dipu runs at 20kms/hr. They start running with Dipu ahead of Raman by 200 metres. At what distance from starting point Raman will catch up with Dipu and how long will he take to catch up?

Solution 3:

Raman will approach Dipu with a relative speed of $24 - 20 = 4$ kms/hr and he will catch up Dipu in a time of,

$ T = \displaystyle\frac{200\text{ metres}}{4\text { km/hr}}=3 \text{ minutes} $

The distance at which Raman will catch up Dipu will be,

$ \text{Catch-up distance} = 3 \text{ mins} \times{24\text{ km/hr}}$

$\hspace{38mm}=\displaystyle\frac{24}{20} \text{ km}=1.2 \text{ km} $

That is, by the time Raman catches up Dipu, running at 20 km/hr Dipu also have run 1 km further in 3 minutes.

Trains running - basic principles

Two trains running in parallel:

The question of relative speed arises when two trains run in parallel. The main quantities that are to be found out in this type of problems are Time to pass,

a second train,
a platform,
a stationary man standing on a platform,
a stationary passenger in another moving train or
a cyclist.

Different cases of two trains moving in parallel are,

Two trains moving in same direction:

When two trains with speeds $S_{1}$ and $S_{2}$ move in parallel and in same direction, the relative speed between the trains is the difference between their speeds, $S_{1} - S_{2}$, assuming $S_{1} > S_{2}$. Train 1 will then pass the train 2 at this relative speed.

If $L_{1}$ and $L_{2}$ are the lengths of the two trains, to pass each other, any of the trains has to traverse a distance of $L_{1} + L_{2}$. So time to pass each other at the relative speed in this case is,

$ \text{Time to pass} = \displaystyle\frac{L_{1} + L_{2}}{S_{1} - S_{2}} $

For example, if the trains move at speeds 60 km/hr and 40 km/hr and and the lengths are 100m and 150m then,

$ \text{Time to pass each other} = \displaystyle\frac{(100 + 150)\text{m}}{(60 - 40)\text{km/hr}}$

$\hspace{50mm}=\displaystyle\frac{250m}{20km/hr}$

$\hspace{50mm}=\displaystyle\frac{250}{20000}hr$

$\hspace{50mm}=45secs$

The first train will completely overtake the second in 45 secs in this case.

Note: It is important to remember that, before final calculation, both the lengths and the speeds have to converted to the same units (1km = 1000m).

If required for simplification, do not forget to convert the time in hours to time in seconds also (1hr = 60mins = 3600secs).

Stages of Two trains passing each other moving in same direction

In two train passing scenario the speedier first train passes the second train in stages.

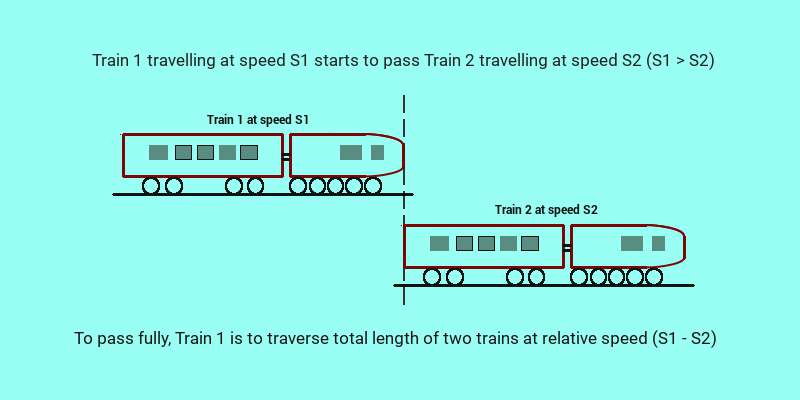
The speedier first train starts passing the slower second train moving in parallel and in same direction. This state of TRAIN PASSING START happens when the nose of the speedier train is aligned with the tail of the slower train from behind.

This is the zero time point of the train passing stages. It is as shown in the following figure. If we consider the above example, the first train moves at 60km/hr while its length is 100m and the second train moves at 40km/hr and its length is 150m. To pass completely, the first train is to traverse $(100+150=250)$m total length of the two trains at the relative speed of $(60-40=20)$km/hr.

Effectively, in train passing, we can imagine that the slower train is standing still and the faster train is crossing the stationary slower train at a relative speed of 20km/hr in this case.

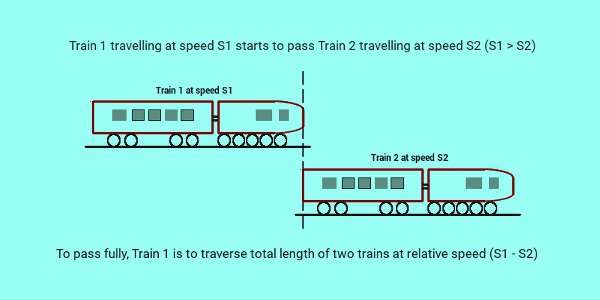
two trains passing each other starts

After a while, the speedier first trains crosses the length of the second train partially. In due time it will leave the second train behind, passing it completely. This partial crossing stage is depicted in the following figure.

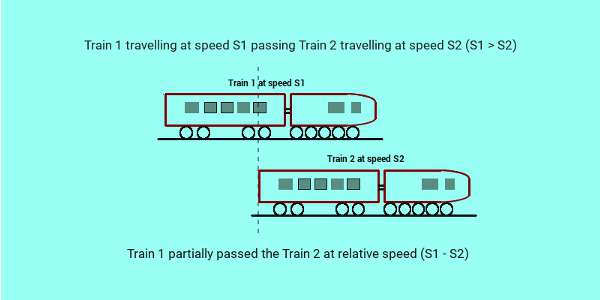
two trains passing each other partially passed

Finally at the last time point of the two train passing scenario, the tail of the speedier first train is aligned with the nose-head of the slower second train, just the opposite of the starting time point state. Next moment the speedier first train will leave the second train completely behind.

This last stage of two train passing scenario is depicted in the following figure. If we consider our example, the first train traveling at 60km/hr has traversed their total length of 250m at their relative speed of 20km/hr in time duration 45secs.

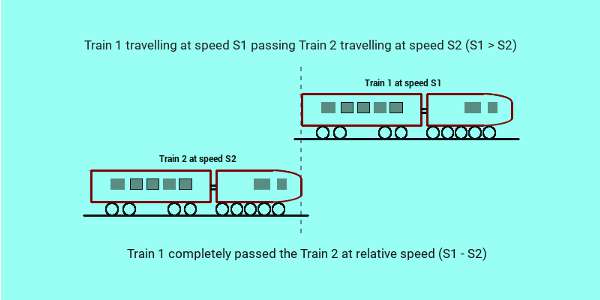
two trains passing each other completely passed

Note: Two objects such as two cars or two racing men moving in parallel and in the same direction catch up and pass each other in exactly the same way.

Two trains moving in opposite direction:

In this case the relative speed will be the sum of their individual speeds, that is,

$\text{Relative speed }= S_{1} + S_{2}$.

In this case the time to pass would obviously be less and is,

$ \text{Time to pass} = \displaystyle\frac{L_{1} + L_{2}}{S_{1} + S_{2}} $

The relative speed is the sum of speeds and this higher value is appearing in the denominator so that time taken will be proportionately less.

With previous example values,

$ \text{Time to pass each other} = \displaystyle\frac{(100 + 150)\text{m}}{(60 + 40)\text{km/hr}}$

$\hspace{50mm}=\displaystyle\frac{250m}{100km/hr}$

$\hspace{50mm}=\displaystyle\frac{250}{100000}hr$

$\hspace{50mm}=9secs$

This makes sense. Earlier the 250m total length was passed at relative speed of 20km/hr. Now the same length is covered but at 5 times the previous speed. As speed and time are inversely proportional, time taken to pass would obviously be one-fifth of 45 secs, that is, 9 secs.

Now we will consider a single moving train:

Various possibilities of the train passing objects are,

A platform: a platform is stationary and so speed of passing will be the train speed, but the length will be the total length of the platform and the train.

For example if the platform is 200m long and the train speed and lengths are 60km/hr and 100m, required time to pass will be,

$\text{Time to pass the platform } $

$= \displaystyle\frac{(100 + 200)\text{m}}{60\text{km/hr}}$

$= \displaystyle\frac{300m}{60000m/hr}$

$=\displaystyle\frac{3600}{200}secs$

$=18secs$.

A stationary man standing on the platform:

A man who is not moving represents a upright line of no width.

Length to pass will be the train length and speed will be the train speed.

For example, the train of length 100m moving at a speed of 60km/hr will pass a man standing on the platform in,

$\text{Time to pass a stationary man} $

$= \displaystyle\frac{100m}{60km/hr}$

$=\displaystyle\frac{100m}{60000m/hr}$

$=\displaystyle\frac{3600}{600}secs$

$=6secs$.

A passenger sitting in another moving train:

In this case, the length to pass will be the length of the passing train, but the speed will be the relative speed of two trains.

It is as if a stick man is running parallel to the first train at the speed of the second train.

For example, first train of length 100m moving at a speed of 60km/hr passing a passenger sitting in a second train of length 150m and moving in parallel at a speed of 40km/hr in opposite direction. What will be the time for the first train to pass the passenger sitting in the second train?

$\text{Time to pass a passenger in 2nd train} $

$= \displaystyle\frac{100m}{(60 + 40)km/hr}$

$=\displaystyle\frac{100m}{100000m/hr}$

$=\displaystyle\frac{3600}{1000}secs$

$=3.6secs$.

Passing other moving objects:

A moving train may pass a man running parallel to it (a man of no width moving at relative speed), or it might well be a case of passing a cyclist moving parallel to the train (the speed of cycle will determine the relative speed, the end to end length of the cycle may be given, or if not given may be assumed to be too small for consideration).

Problem examples