SSC CGL level Solution Set 36, Geometry 4

Quick solutions to inradius circumradius circle questions in SSC CGL Set 36

Learn to solve 10 inradius circumradius questions for SSC CGL Set 36 in 15 minutes using basic and advanced geometry concepts.

For best results take the test first at,

SSC CGL level Question Set 36, Geometry 4.

---

Learn to solve inradius circumradius questions for SSC CGL Set 36 - answering was time 15 mins

Problem 1.

The radius of the circumcircle of a right angled triangle is 15 cm and the radius of the inscribed circle is 6 cm. Then the length of the three sides (in cm) are,

1. 30, 24, 25
2. 24, 36, 20
3. 18, 24, 30
4. 30, 40, 41

Solution 1.

The following figure describes the problem,

In any geometric problem it is usually gainful to draw a quick sketch depicting the problem. When we sketched the problem figure above and analyzed it briefly we recognized that,

• the triangle is a right angled triangle -- from problem definition
• the triangle has one side $2\times{15}=30$ cms long -- from the figure, and thirdly from the figure again,
• the side of length 30 cm is in fact the hypotenuse of the right angled triangle and consequently the largest of the three sides.

At this point we decided to test the values in the choices using the three pieces of information.

This is direct use of free resource use principle where free resource is the set of values in the given choices.

30 as the largest value appears only in the two choice sets, 30, 24, 25 and 18, 24, 30. So our solution is one of these two sets.

Now we test these two sets to see whether the sets follow Pythagoras theorem so that sum of squares of two smaller values equals the square of the third largest value, that is, 900.

Avoiding the test by squaring that takes more time, we resort to the faster test of units digit behavior analysis,

For the sum of squares of two numbers to equal 900 which is even, the units digits of the two numbers must both be even or both be odd.

Only the value set 18, 24, 30 satisfies this basic number system concept and so is the solution.

For confirmation you may test this set for Pythagoras relation to find that it actually satisfies the Pythagoras relation,

$18^2 + 24^2 = 324 + 576 = 900 = 30^2$.

Answer: c: 18, 24, 30.

Key concepts used: Visualization of the largest side of the right angled triangle as the hypotenuse of length 30 cm -- use of free resource principle and testing the given information against the free resources of the choice value sets -- 30 cm as the largest side length eliminates two sets of values -- Pyhthagoras theorem -- basic number system concept of units digit behavior analysis eliminates one of the two remaining sets to home in to the solution.

Note: The incircle information turns out to be superfluous.

Problem 2.

A chord $AB$ of a circle of radius $(\sqrt{3} + 1)$ cm touches a second concentric circle of radius $(\sqrt{3} - 1)$ cm. The length of $AB$ (in cm) is,

1. $2\sqrt[4]{3}$
2. $4\sqrt{3}$
3. $8\sqrt{3}$
4. $4\sqrt[4]{3}$

Solution 2.

The following figure describes the problem.

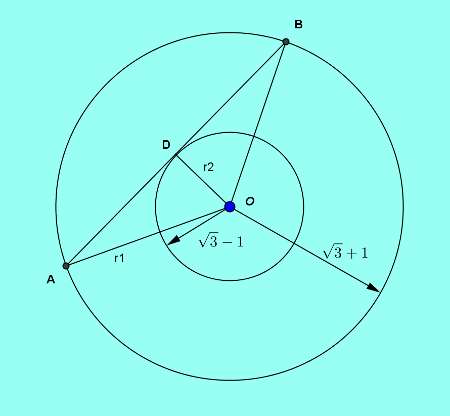
In the larger outer circle, the chord $AB$ is bisected by the perpendicular $OD$ from centre $O$. But $AB$ also being the tangent to the inner concentric circle, the radius of the smaller inner circle $OD$ is the same perpendicular on $AB$ at $D$ the point of tangency. 

This can be classified as a rich concept set of Concentric Circle concepts. In any pair of concentric circles these conclusions are true.

By Pyhthagoras theorem in $\triangle AOD$ then,

$AD^2 = AO^2 - OD^2$

$\hspace{10mm}=r_1^2 - r_2^2$

$\hspace{10mm}=(\sqrt{3} + 1)^2 - (\sqrt{3} - 1)^2$

$\hspace{10mm}=[(\sqrt{3} + 1) + (\sqrt{3} - 1)][(\sqrt{3} + 1) - (\sqrt{3} - 1)]$

$\hspace{10mm}=4\sqrt{3}$.

So, $AD=2\sqrt[4]{3}$

Thus the required length of the chord is,

$AB = 2AD= 4\sqrt[4]{3}$ cm

Answer: d: $4\sqrt[4]{3}$.

Key concepts used: Rich concept set of concentric circle concepts -- Pythagoras theorem -- use of basic algebraic concept, $a^2-b^2 = (a+b)(a-b)$ to speed up calculation.

Problem 3.

If the inradius of an equilateral triangle be 5 cm, its circumradius (in cm) is,

1. 10
2. 15
3. 25
4. 30

Solution 3.

The problem is depicted in the figure below.

AB, BC and CA are the three sides of the equilateral triangle where O is its circumcentre and OA, OB and OC its circumradii. Being an equilateral triangle (with AB=AC), the line AOD through vertex A and the centre O is the perpendicular bisector of BC (which is a chord of the larger circle) and so is a median of the triangle. Similarly the other two medians from the two vertices B and C also pass through the centre O (because AB=BC=CA) which is then not only the circumcentre, but also the coincident centroid.

By basic tangent concepts, the intercepts of the two adjacent tangents to the inner concentric circle from B, $BE$ and $BD$ are equal. But as chords $BC=AB$, the radii of the inner circle $OD$ and $OE$ are not only perpendicular to the chords but also bisect the chords of the larger circle, $BC$ and $AB$. By the same reasoning, radius $OF$ of the inner circle is the perpendicular bisector of the third side $AC$ that is also tangent to the inner circle.

Thus the smaller concentric circle is the inscribed circle and $O$ is indeed the coincident point of three important points of the equilateral $\triangle ABC$, the circumcentre, the centroid and the incentre.

This set of concepts can be classified as the rich concept set for equilateral triangles. To state formally,

For any equilateral triangle, the circumcentre, the centroid and the incentre will be coincident.

Lastly, $O$ being the centroid, and $AOD$ being a median it is divided at centroid O in a ratio of 2 : 1, that is, the length of AO is double the length of OD which is 5 cm. Thus the circumradius is $2\times{5} = 10$ cm long.

This final result can again be classified as a rich geometric concept derived from basic concepts. To state this concept formally,

For any equilateral triangle, its circumradius is double the length of its inradius.

Answer: a: 10.

Key concepts used: Rich concept of coincidence of centroid, incentre and circumcentre of an equilateral triangle -- median dissection ratio concept -- rich concept of relation between lengths of inradius and circumradius of an equilateral triangle.

Problem 4.

In isosceles $\triangle ABC$ right-angled at B, DP and DQ are two perpendiculars dropped from a point D inside the triangle on the two sides AB and AC such that P and Q lie on AB and AC respectively. If $AP = a$ cm, $AQ=b$ cm and $\angle BAD=15^0$, then $\sin 75^0$ is,

1. $\displaystyle\frac{a}{2b}$
2. $\displaystyle\frac{2b}{\sqrt{3}a}$
3. $\displaystyle\frac{\sqrt{3}a}{2b}$
4. $\displaystyle\frac{2a}{\sqrt{3}b}$

Solution 4.

The following figure depicts the given problem.

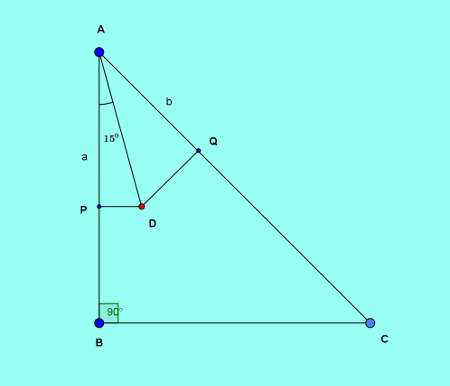
In right angled isosceles $\triangle ABC$, $AB=BC$ and $AC$ is the hypotenuse. Thus both the angles $\angle BAC$ and $\angle ACB$ are $45^0$.

In $\triangle APD$ right-angled at P, $\angle PAD=15^0$ and so, $\angle ADP=75^0$.

Thus, $\sin 75^0 = \displaystyle\frac{AP}{AD} = \frac{a}{AD}$.

Turning our attention to $\triangle AQD$ right-angled at Q we have,

$\angle DAQ = \angle BAC - \angle PAD = 45^0 - 15^0 = 30^0$.

So, $\displaystyle\frac{AQ}{AD} = \frac{b}{AD} = \cos 30^0 = \frac{\sqrt{3}}{2}$,

Or, $\displaystyle\frac{1}{AD} = \frac{\sqrt{3}}{2b}$

Substituting in the first equation,

$\sin 75^0 = \displaystyle\frac{a}{AD} = \frac{\sqrt{3}a}{2b}$.

In this way the unknown length of $AD$ is eliminated by the use of two given known variable values.

Answer: c: $\displaystyle\frac{\sqrt{3}a}{2b}$.

Key concepts used: Basic geometric concepts -- basic trigonometric concepts -- deductive reasoning.

Note: It is interesting to observe that for solving geometric problems, occasionally you may need to use basic trigonometric concepts just as we have used basic number system concepts and basic algebraic concepts earlier.

Problem 5.

Sides of a right-angled triangle are in the ratio 4 : 5 : 6. If the in-radius of the triangle is 3 cm, the altitude of the triangle with base as the largest side is,

1. 7.5 cm
2. 6 cm
3. 8 cm
4. 10 cm

Solution 5.

The figure depicting the problem is shown below.

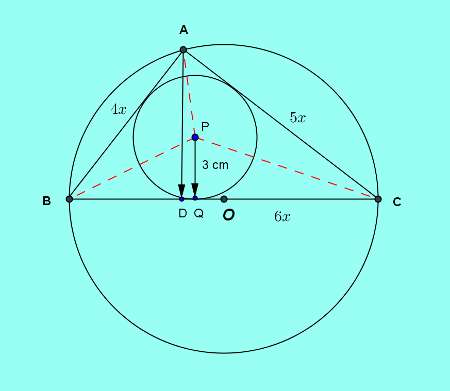
By the basic ratio concepts we introduce the canceled out HCF of the three side lengths as a factor to transform the given ratio to, $4x : 5x : 6x$. Given is the length of inradius $PQ=3$ cm and to be evaluated, the measure of the height of $AD$ with the largest side $BC$ as the base.

To relate length of side with height of a triangle, the best measure is the area of a triangle. With this key idea, when we examine the figure closely we could see the main triangle composed of three smaller triangles, $\triangle OBC$ , $\triangle OAB$, and $\triangle OAC$.

Putting this idea into practice we have the equation of areas of triangles as,

$\frac{1}{2}BC\times{AD} = \frac{1}{2}3(AC + AB + BC)$

Or, $6xAD=3(4x +5x+6x)$

Or, $AD = \frac{15}{2} = 7.5$

Answer: a: 7.5 cm.

Key concepts used: Basic ratio concepts -- introduction of the canceled out HCF as a factor to all the three ratio terms -- choosing area of a constituent triangles as the mechanism to relate the heights with base lengths in triangles -- putting the idea into practice as the equation of areas -- elimination of the unknown HCF to get the desired measure of the height.

Problem 6.

Inside a square $\square ABCD$, $\triangle BCE$ is an equilateral triangle. If CE and BD intersect at O, then $\angle BOC$ is equal to,

1. $75^0$
2. $60^0$
3. $90^0$
4. $120^0$

Solution 6.

The following figure represents the problem.

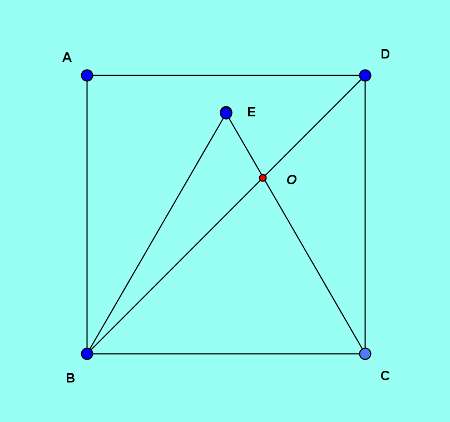
As BD is the diagonal of a square it bisects the $90^0$ angle at B and so, $\angle CBO=45^0$.

Again in the equilateral $\triangle BEC$, $\angle CBE=60^0$.

So in the $\triangle BEO$, $\angle OBE = 60^0 - 45^0 = 15^0$ and $\angle BEO=60^0$. Thus the third angle $\angle BOE=180^0 - 60^0 - 15^0=105^0$.

Thus in the intersection of two straight lines at the point O,

$2\times{\angle BOC} = 360^0 - 2\times{105^0}=150^0$.

And so finally,

$\angle BOC = 75^0$.

Answer: a: $75^0$.

Key concepts used: Concept of angles in a square and the fact that a diagonal in a square bisects the $90^0$ angle -- equilateral triangle internal angle as $60^0$ -- evaluation of one of the angles at the cross-point intersection of two straight lines O from sum of angles in a triangle -- concept of relation of four angles at the intersection of two straight lines, all basic geometric concepts homing in to the desired angle.

Problem 7.

$ABCD$ is a rectangle where the ratio of the lengths $AB$ and $BC$ is 3 : 2. If $P$ is the midpoint of $AB$ then the value of $\sin \angle CPB$ is,

1. $\displaystyle\frac{3}{5}$
2. $\displaystyle\frac{3}{4}$
3. $\displaystyle\frac{4}{5}$
4. $\displaystyle\frac{2}{5}$

Solution 7.

The following figure represents the problem.

For convenience of calculation, with the ratio of AB : BC = 3: 2, we will assume $AB=6x$ and $BC=4x$ eliminating the need of 2 in the denominator by halving AB.

So in right-angled $\triangle CPB$,

$CP^2 = PB^2 + BC^2 = 9x^2 + 16x^2 = 25x^2$,

Or, $CP=5x$,

Or, $\sin \angle CPB = \displaystyle\frac{BC}{CP} = \frac{4x}{5x}=\frac{4}{5}$.

Answer: c: $\displaystyle\frac{4}{5}$.

Key concepts used: Basic ratio concepts -- basic trigonometric concepts.

Alternate solution:

By introducing the canceled out HCF in the ratio terms of 3 : 2 we get the actual values of $AB$ and $BC$ as $3x$ and $2x$. We have then, P being the midpoint of $AB$, $PB=\displaystyle\frac{3x}{2}$.

Let us further assume $\angle CPB = \theta$ for convenience. So the desired quantity,

$\sin \angle CPB=\sin \theta$, where we have the known value of,

$\tan \theta = \displaystyle\frac{BC}{PB} = \frac{2x}{\displaystyle\frac{3x}{2}} = \frac{4}{3}$,

Or, $\cot \theta = \displaystyle\frac{3}{4}$,

Or, $cosec^2 \theta = 1 + \cot^2 \theta = \displaystyle\frac{25}{16}$

Or, $cosec \theta = \displaystyle\frac{5}{4}$,

Or, $\sin \theta = \sin \angle CPB = \displaystyle\frac{4}{5}$.

This is an example of application of Many ways technique.

Problem 8.

The distance between two parallel chords of length 8 cm each in a circle of diameter 10 cm is,

1. 7 cm
2. 6 cm
3. 5.5 cm
4. 8 cm

Solution 8.

The following is a depiction of the problem graphically.

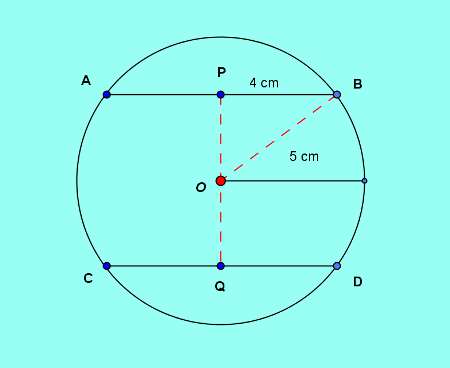
$AB$ and $CD$ are the two chords of length 8 cm each on opposite sides of the centre $O$ separated by a distance of $PQ$.

In right-angled $\triangle OPB$, $OB=5$ cm, being a radius and $P$ being the mid-point of the chord $AB$ bisected by the perpendicular $OP$ from the centre, $PB=4$ cm which is half of chord length of 8 cm.

Thus by Pythagoras theorem,

$OP^2 = OB^2 - PB^2 = 5^2 - 4^2 = 9$,

Or, $OP=3$,

Or, separation between two chords,

$PQ=2OP=6$ cm.

Answer: b: 6 cm.

Key concepts used: Visualization -- chord bisection by perpendicular from centre -- Pythagoras theorem.

Problem 9.

A square $ABCD$ is inscribed in a circle of unit radius. Semicircles are described externally on each side with the side as the diameter. The area of the region bounded by the semicircles and the circle is,

1. 1 sq unit
2. 2.5 sq units
3. 1.5 sq units
4. 2 sq units

Solution 9.

The following figure represents the problem description.

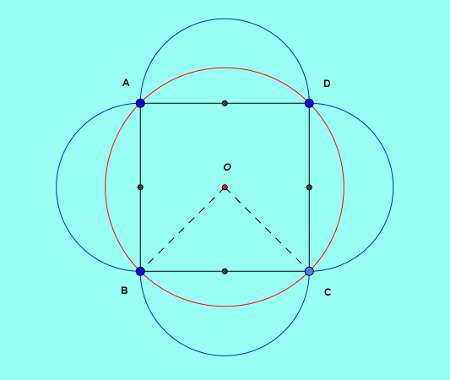
To find out the area in four similar parts between the outer boundary of semicircles in blue and the inner boundary of the circle in red. This desired area is,

Area bounded by the circle and the semicircles = Area of semicircles + Area of square - Area of circle.

OB and OC are half-diagonals of the square and thus each bisect the corner angle of $90^0$ so that the angle $\angle BOC=90^0$. In $\triangle BOC$ then applying Pythagoras theorem,

$BC^2 = OB^2 + OC^2 = 2$

Or, $BC = \sqrt{2}$.

So, $\text{Area of square} = 2$.

$\text{Area of the circle} = {\pi}r^2=\pi$, as the radius is unity.

As $BC=\sqrt{2}$ is the diameter, the radius of each semicircle is $\frac{1}{2}\sqrt{2}=\frac{1}{\sqrt{2}}$ and its area, $\frac{1}{4}\pi$.

Thus $\text{Area of the four semicircles} = 4\times{\frac{1}{4}}{\pi}=\pi$.

So the desired area = $\pi + 2 - \pi=2$ sq units.

Answer: d: 2 sq units.

Key concepts used: Pythagoras theorem -- areas of squares and circles -- area identification from problem definition.

Problem 10.

A, B and C are three points on a circle such that angles subtended by the chords AB and AC at the centre are non-overlapping $90^0$ and $110^0$ respectively. $\angle BAC$ is then equal to,

1. $80^0$
2. $90^0$
3. $100^0$
4. $70^0$

Solution 10.

The following is the figure relevant to the problem.

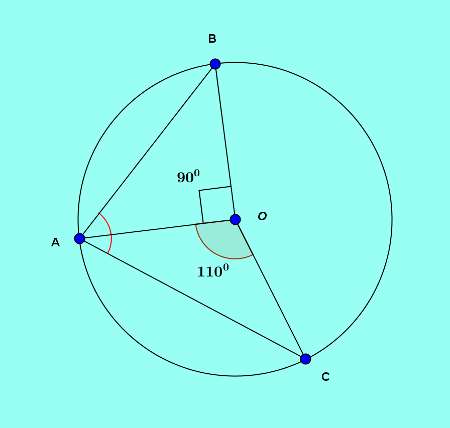
From the figure,

$\angle BOA + \angle COA = 90^0 + 110^0 = 200^0$.

So, $\angle COB = 360^0 - 200^0=160^0$.

This angle is subtended by the arc CB at the centre and so it should be double the angle held by the arc on the periphery which is the desired angle,

$\angle BAC = \frac{1}{2}160^0 = 80^0$.

Answer: a: $80^0$.

Key concepts used: Total angle held at the centre -- angle subtended by an arc at the centre and the periphery.

Alternate solution:

Being radii, $OA=OB$ and in isosceles $\triangle OAB$ with apex $\angle AOB=90^0$,

$\angle OAB = \frac{1}{2}90^0=45^0$.

Similarly in isosceles $\triangle OAC$, with apex $\angle AOC=110^0$,

$\angle OAC = \frac{1}{2}(180^0 - 110^0) = 35^0$.

So, desired $\angle BAC = \angle OAB + \angle OAC = 45^0 + 35^0 = 80^0$.

Evaluate the two methods of solution and choose what you think best. This is another application of Many ways technique.

---

Guided help on Geometry in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on Geometry in Suresolv, follow the guide,

Suresolv Geometry Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II and Other Competitive exams.

The guide list of articles includes ALL articles on Geometry and relevant topics in Suresolv and is up-to-date.

---