Basic and Rich Geometry Concepts Part 8, Internal Angle bisectors and Segment Ratios at Incentre of a triangle

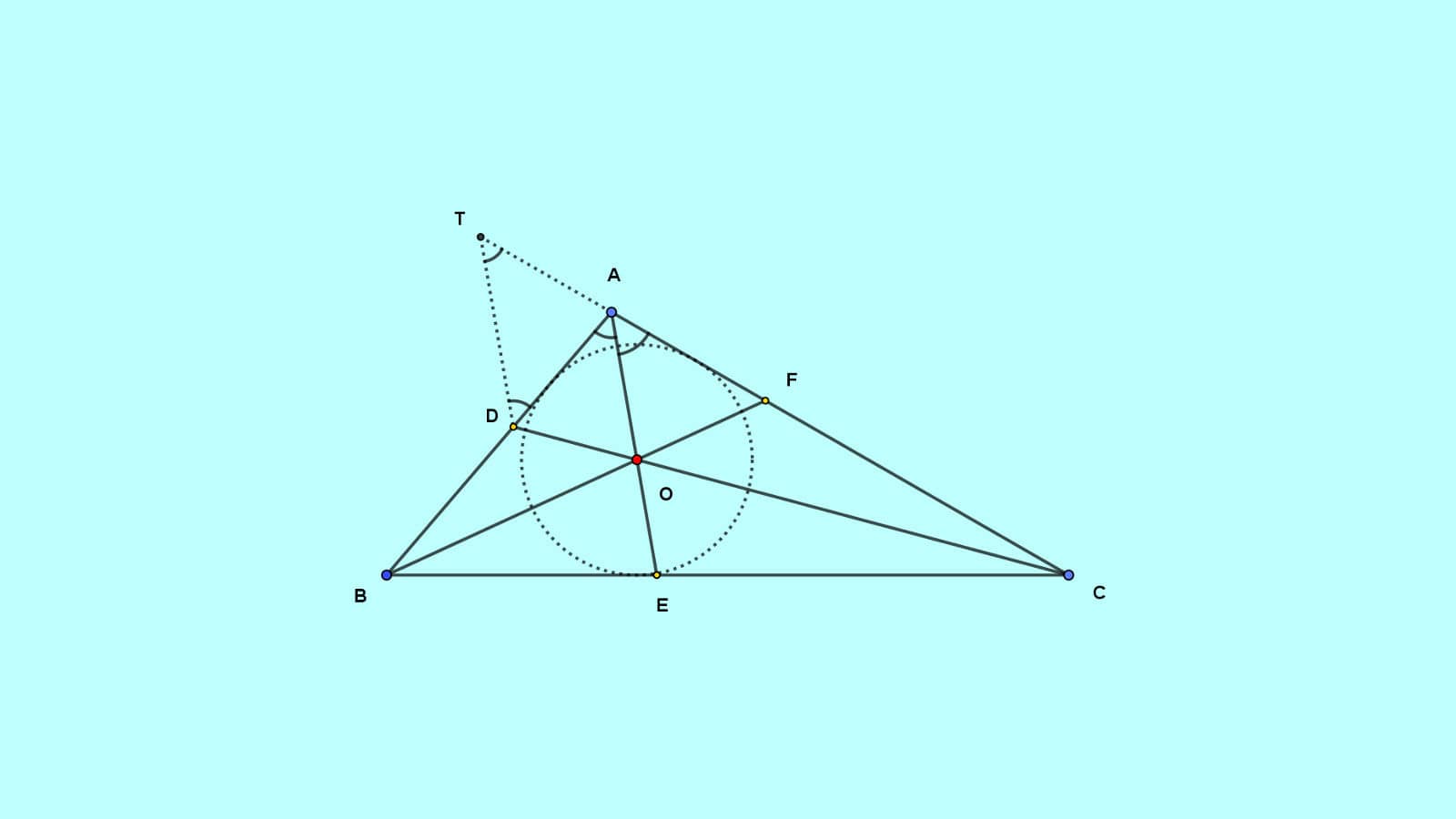
Incenter of a triangle and Angle bisectors: Segmentation ratios at the incenter

Incenter of a triangle and Angle bisectors: Segmentation of the opposite side by an angle bisector and Segmentation of the angle bisectors at the Incenter.

Content sections are,

1. Incenter of a triangle: The center of the circle inscribed in a triangle.
2. Incenter of a triangle and angle bisectors: Internal angle bisectors meet at the incenter of a triangle: Concept and proof.
3. Internal angle bisector segments opposite side of a triangle in the ratio of the two adjacent sides: Concept and proof.
4. Incenter angle bisector ratio of a triangle: Ratio of internal angle bisector segments at incenter related to ratio of sides.
5. Problem example on use of incenter angle bisector ratio solved using segmentation ratio of internal angle bisectors.

You may click on any of the above section links to move on directly to the section and return by clicking on browser back button.

Incenter of a triangle

Incenter of a triangle is the center of the circle inscribed in it. The center O of the circle inscribed in the $\triangle ABC$ in figure below is the incenter of the triangle.

P, Q and R are the tangent points of the inscribed circle and AB, BC and CA are the three sides of the $\triangle ABC$ tangent to the inscribed circle at these points.

Perpendiculars to the sides AB, BC and CA at these points are OP, OQ and OR.

These meet at point O, center of the incircle. These are the radii of the incircle.

This describes the main elements we will use now.

The incircle with center O is defined and drawn then as,

The circle drawn inside a triangle to which each of the three sides are tangents to the inscribed circle. The center of this circle is the incenter of the triangle.

Incenter of a triangle and Angle bisectors

AO, BO and CO are the bisectors of the internal angles, $\angle A$, $\angle B$ and $\angle C$. These are the internal angle bisectors.

Basic property of the internal angle bisectors is,

The three internal angle bisectors of a triangle meet at a single point. This is the incenter of the triangle.

Proof of internal angle bisectors meeting at incenter

The same graphic as above we will use for the proof.

Radii OP and OR are perpendiculars to tangent sides AB and AC in triangles $\triangle AOP$ and $\triangle AOR$.

So, $\angle APO=\angle ARO=90^0$.

The two triangles are then right-angled. In the two triangles, hypotenuse AO is common and radii $OR=OP$. So the third pair of sides also equal, $AP=AR$.

Three pairs of corresponding sides are then equal in  $\triangle AOP$ and $\triangle AOR$. So these are congruent to each other.

It follows, all the corresponding angles of the two triangles are equal.

So,

$\angle PAO=\angle RAO$.

That is, AO is the internal angle bisector of $\angle A$ of $\triangle ABC$.

And the other two line segments, BO and CO also are the internal angle bisectors of the angles $\angle B$ and $\angle C$.

So the point O has two roles. It is,

1. center of the circle inscribed in $\triangle ABC$, and,
2. Concurrent intersection point of three internal angle bisectors of $\triangle ABC$.

An internal angle bisector segments the opposite side in the ratio of the two adjacent sides: Concept and proof

The first incenter segmentation ratio relation in a triangle states,

Internal angle bisector segments opposite side in two segments. Ratio of these two segments is equal to the ratio of two sides containing the bisected angle.

For explaining the proof of the result, we will use the following graphic.

By this segmentation result in $\triangle ABC$,

$\displaystyle\frac{BE}{CE}=\frac{AB}{AC}$.

Let us see how this is true.

Draw a line parallel to the internal angle bisector AE passing through B. It meets side CA extended at S.

As in $\triangle BCS$, SB is parallel to AE, the two triangles $\triangle BCS$ and $\triangle ECA$ are similar.

Reason: $\angle C$ common and the other two pairs of angles also equal as $SB || AE$. This satisfies A-A-A similarity condition.

As a result, ratio of corresponding sides are equal,

$\displaystyle\frac{SC}{AC}=\frac{BC}{CE}$.

Subtract 1 from both sides with the result,

$\displaystyle\frac{SC-AC}{AC}=\frac{BC-CE}{CE}$,

Or, $\displaystyle\frac{SA}{AC}=\frac{BE}{CE}$.

But as $SB || AE$,

$\angle SBA=\angle BAE$, and,

$\angle ASB=\angle CAE$.

So with two parts of bisected $\angle A$, $\angle BAE=\angle CAE$,

$\angle SBA =\angle ASB$.

As a result, $\triangle ASB$ is isosceles, and, $SA = AB$.

The segment ratio takes its final form then as,

$\displaystyle\frac{BE}{CE}=\frac{AB}{AC}=\frac{c}{b}$, using the short form of naming the sides of a triangle.

In the same way,

$\displaystyle\frac{CF}{AF}=\frac{BC}{AB}=\frac{a}{c}$, and,

$\displaystyle\frac{AD}{BD}=\frac{AC}{BC}=\frac{b}{a}$.

Incenter angle bisector ratio: Ratio of segments of an internal angle bisector at the incenter in terms of sides of the triangle

Specifically incenter ratio relation states,

Ratio of segments of an internal bisector made at incenter equals ratio of sum of adjacent sides to opposite side of triangle.

With respect to $\triangle ABC$ in the following diagram, this relation is expressed as,

$\displaystyle\frac{CO}{OD}=\frac{AC+BC}{AB}=\frac{a+b}{c}$.

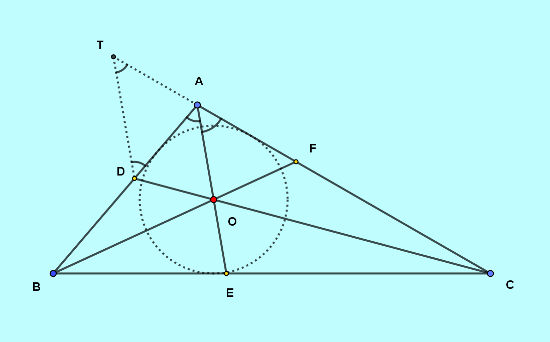
Let us go through the proof of this result.

Draw a line segment DT parallel to AO that meets the extended side CA at T.

By the construction the two triangles, $\triangle CTD$ and $\triangle CAO$ are similar. So ratio of the corresponding sides of the two triangles are equal. For instance,

$\displaystyle\frac{CD}{CO}=\frac{CT}{AC}$.

Subtract 1 from both sides with the result,

$\displaystyle\frac{CD-CO}{CO}=\frac{CT-AC}{AC}$,

Or, $\displaystyle\frac{OD}{CO}=\frac{AT}{AC}$.

$\angle DAO= \angle CAO$ as these are two equal bisected parts of $\angle CAD$. Also DT and AO are parallel. So,

$\angle ADT=\angle DAO$ and $\angle ATD= \angle CAO$.

We have,

$\angle ADT=\angle ATD$ and as a result $\triangle ADT$ isosceles.

It follows, sides, $AT=AD$.

Substitute this result into the segment relation to get the result,

$\displaystyle\frac{OD}{CO}=\frac{AD}{AC}$,

Or, $\displaystyle\frac{CO}{OD}=\frac{b}{AD}$.

In the RHS, $AD$ being is segment of the side $AB$. It is to be expressed in terms of other two sides so that the RHS is wholly in terms of sides of the triangle.

To do this equate $\displaystyle\frac{b}{AD}$ with an expression in terms of only the sides of the triangle.

Recall our earlier result of segmentation at the opposite side result,

$\displaystyle\frac{AD}{BD}=\frac{b}{a}$.

Invert this to get,

$\displaystyle\frac{BD}{AD}=\frac{a}{b}$.

Add 1 to both sides of the equation,

$\displaystyle\frac{AD+BD}{AD}=\frac{a+b}{b}$,

Or, $\displaystyle\frac{AB}{AD}=\frac{c}{AD}=\frac{a+b}{b}$,

Or, $\displaystyle\frac{b}{AD}=\frac{a+b}{c}$.

Substitute this desired result into the segmentation result and eliminate side segment AD.

The final desired form of segmentation ratio equality at incenter is then,

$\displaystyle\frac{CO}{OD}=\frac{b}{AD}=\frac{a+b}{c}$.

The ratio of the segments of internal angle bisector at incenter equals the ratio of the sum of adjacent sides and the opposite side.

Similarly, we get the other two segmentation results for angle bisectors at incenter as,

$\displaystyle\frac{AO}{OE}=\frac{b+c}{a}$, and,

$\displaystyle\frac{BO}{OF}=\frac{c+a}{b}$.

This is the final form of the advanced concept of incenter ratio.

It is often used for solving difficult Geometry problems quickly in a few simple steps.

We will solve such an example problem now to show how it is possible to solve a difficult problem quickly by this concept.

Problem example on use of incenter angle bisector ratio of segments: Application on incenter angle bisector ratio

Question: In the given figure, O is the incenter of $\triangle ABC$. If $\displaystyle\frac{AO}{OE}=\frac{5}{4}$ and $\displaystyle\frac{CO}{OD}=\frac{3}{2}$, what is the value of $\displaystyle\frac{BO}{OF}$?

1. $\displaystyle\frac{19}{14}$
2. $\displaystyle\frac{19}{7}$
3. $\displaystyle\frac{38}{7}$
4. $\displaystyle\frac{38}{17}$

Solution to example problem

Given,

$\displaystyle\frac{AO}{OE}=\frac{b+c}{a}=\frac{5}{4}$,

Or, $\displaystyle\frac{a+b+c}{a}=\frac{9}{4}$, adding 1 to both sides.

Inverting,

$\displaystyle\frac{a}{a+b+c}=\frac{4}{9}$.

The other given relation similarly is,

$\displaystyle\frac{CO}{OD}=\frac{a+b}{c}=\frac{3}{2}$,

Or, $\displaystyle\frac{a+b+c}{c}=\frac{5}{2}$, adding 1 to both sides of the equation.

Inverting in the same way as before,

$\displaystyle\frac{c}{a+b+c}=\frac{2}{5}$.

Add these two intermediate results,

$\displaystyle\frac{c+a}{a+b+c}=\displaystyle\frac{2}{5}+\displaystyle\frac{4}{9}=\displaystyle\frac{38}{45}$.

Invert this result again,

$\displaystyle\frac{a+b+c}{c+a}=\frac{45}{38}$.

Subtract 1 from both sides,

$\displaystyle\frac{b}{c+a}=\frac{7}{38}$.

Inverting we get the solution.

$\displaystyle\frac{c+a}{b}=\frac{BO}{OF}=\frac{38}{7}$.

Answer: Option c: $\displaystyle\frac{38}{7}$.

This is use of rich concept of segmentation ratio of internal angle bisectors at incenter and use of smart algebraic techniques.

---

Guided help on Geometry in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on Geometry in Suresolv, follow the guide,

Suresolv Geometry Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II and Other Competitive exams.

The guide list of articles includes ALL articles on Geometry and relevant topics in Suresolv and is up-to-date.

---

←   →