Geometry basic concepts Part 2, Quadrilaterals Polygons Squares

Quadrilateral basic concepts on Polygons Squares Parallelogram

Quadrilateral basic Concepts: Properties of a quadrilateral, polygons, squares, parallelograms, rhombus, cyclic quadrilateral with examples and exercise.

Contents are on Geometry basics,

1. Types of quadrilaterals: Parallelogram, Rectangle, Rhombus, Square.
2. Cyclic quadrilateral.
3. Internal and external angles of a polygon.
4. Exercise questions with answer.

To refresh basic geometry concepts, refer to the first concept article on Points lines and triangle.

And to know about the most important geometric shape of cicrles refer to the third concept article on Circles.

What is a quadrilateral

Let us start this session by showing you what a quadrilateral is.

A closed shape on a plane bounded by four straight line edges connected at four vertices is a quadrilateral. Example:

Properties of a quadrilateral:

• A quadrilateral is an enclosed shape on a plane with four straight line sides connected at four vertices or corners without any side crossing any other.
• Sum of its four interior angles is $360^0$.

Parallelogram

When two opposite sides of a quadrilateral are parallel, and the lengths equal, the shape is a parallelogram.

• The opposite sides $AB || DC$ and $AB=DC$, as also, $AD || BC$ and $AD=BC$.
• The opposite internal angles $\angle A=\angle C$ and $\angle B=\angle D$.
• Each of the diagonals divide the parallelogram into two congruent triangles: $\triangle ABC\equiv\triangle ADC$ and $\triangle DAB\equiv\triangle DCB$.

Rectangle

When the angles of a parallelogram each becomes a right angle, we call the shape as a rectangle.

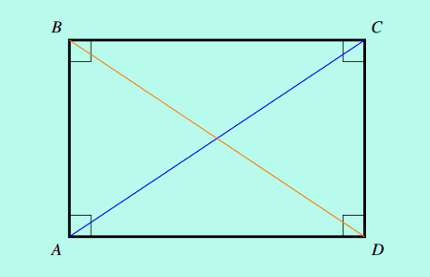
• The opposite sides $AB || DC$ and $AB=DC$, as also, $AD || BC$ and $AD=BC$.
• All internal angles $\angle A=\angle B=\angle C=\angle D=90^0$.
• Each of the diagonals divides the rectangle into two congruent triangles: $\triangle ABC\equiv\triangle ADC$ and $\triangle DAB\equiv\triangle DCB$.
• All four triangles created by the two diagonals are congruent to each other: $\triangle ABC\equiv\triangle ADC\equiv\triangle DAB\equiv\triangle DCB$.

Rhombus

When the four sides of a parallelogram are equal we call the shape a Rhombus.

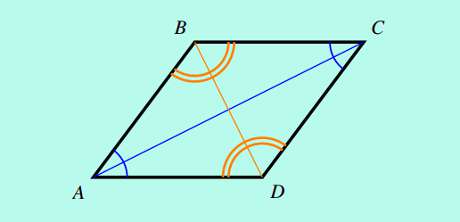
• Opposite sides are parallel: $AB || DC$ and $AD || BC$ and all sides are equal $AB=BC=CD=DA$.
• The opposite internal angles $\angle A=\angle C$ and $\angle B=\angle D$.
• Each of the diagonals divides the parallelogram into two congruent triangles: $\triangle ABC\equiv\triangle ADC$ and $\triangle DAB\equiv\triangle DCB$.
• Additionally, the diagonals bisect the opposite angles: $\angle CAD=\angle CAB=\angle ACB=\angle ACD$ and $\angle DBA=\angle DBC=\angle BDA=\angle BDC.$
• Lastly, the diagonals are perpendicular to each other and bisect each other.

Square

When all the sides of a quadrilateral are equal as well as all the angles are right angles, the shape we call a square.

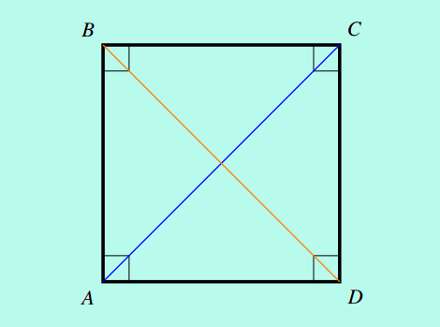
• Opposite sides are parallel: $AB || DC$ and $AD || BC$, as also all four sides are equal: $AB=DC=AD=BC$.
• All angles $\angle A=\angle B=\angle C=\angle D=90^0$.
• The diagonals divide the square into four congruent triangles: $\triangle ABC\equiv\triangle ADC\equiv\triangle DAB\equiv\triangle DCB$.
• The diagonals bisect the opposite angles: $\angle CAD=\angle CAB=\angle ACB=\angle ACD$ and $\angle DBA=\angle DBC=\angle BDA=\angle BDC.$ All of these bisected angles are equal and are $45^0$.
• The diagonals are perpendicular bisecors to each other.
• The diagonals divide the area of the square into four equal parts.

Cyclic quadrilateral

When four vertices of a quadrilateral lie on the circumference of a circle, it is called a cyclic quadrilateral. One of the main properties of a cyclic quadrilateral is its opposite angles summing up to an angle measure of $180^0$. We will see why it happens in our next session.

Sum of internal angles of a polygon

Sum of internal angles of a triangle is $180^0$, of a quadrilateral is $360^0$, but what is the sum of internal angles of a polygon with $n$ sides?

This sum is $(n - 2)\pi$.

Let us take a hexagon to understand this.

In a hexagon there are six sides and six vertices. Taking a regular hexagon of equal sides, we can draw a circumscribed circle on which all the six vertices will lie. If these vertices are connected with the center of the circle inside the hexagon, we get six triangles.

Sum of the angles of these six triangles is $n\pi=6\pi$ and the sum of the angles at the center is $2\pi$.

Thus,

Sum of the base angles of the $n$ triangles at the six vertices of the hexagon

$=$ Sum of the angles of the triangles $-$ Sum of the angles at the vertices of the triangles or center of the circle

$= n\pi - 2\pi$

$=(n - 2)\pi$.

The number of sides in the hexagon, $n=6$ and sum of its internal angles,

$ \angle A + \angle B + \angle C + \angle D + \angle E + \angle F=(n - 2)\pi=4\pi=720^0 $,

as total sum of the angles of the six triangles $\triangle AOB$, $\triangle BOC$ and so on is $n\pi$ or $6\pi$, total sum of the vertex angles, $\angle AOB$, $\angle BOC$ and so on at center $O$ is $2\pi$ and the sum of internal angles of the hexagon is the difference of the two, that is, $n\pi - 2\pi=(n - 2)\pi$.

If each internal angle = $a_i$, then the same sum of internal angles can be expressed as,

$na_i = \pi(n - 2)$.

Sum of external angles of a regular polygon

If $a_e$ is each of the external angles of a regular polygon, the total will be = $na_e$.

By definition, an individual external angle is the difference between $\pi$ and the internal angle, that is, $a_e = \pi - a_i$.

So relating to number of sides, the total of external angles is,

$na_e = n(\pi - a_i) $

$\hspace{8mm}= n\pi - na_i $

$\hspace{8mm}= n\pi - \pi(n - 2)$

$\hspace{8mm}=2\pi$.

Whatever be the number of sides, the total of the external angles always sum up to $2\pi$.

This happens because as the number of sides increases, the amount of an internal angle also goes on increasing and so the external angle goes on decreasing.

Can you say what the limit of internal angle increase is?

The limit is $180^0$ which the internal angle will strive to reach but will never reach. If it reaches $180^0$, the space of the polygon becomes an unbounded straight line.

Let us end the session with a problem exercise set for you.

---

Problem exercise

The recommended time limit is 18 minutes

Problem 1.

In a cyclic quadrilateral $ABCD$, side $AB$ is extended to $E$ so that $BE = BC$. If $\angle ADC=70^0$ and $\angle BAD=95^0$ then $\angle DCE$ is,

1. $140^0$
2. $165^0$
3. $120^0$
4. $110^0$

Problem 2.

The ratio between the number of sides of two regular polygons is $1 : 2$ and the ratio between their interior angles is $2 : 3$. The number of sides of the polygons are respectively,

1. 5, 10
2. 6, 12
3. 7, 14
4. 4, 8

Problem 3.

The length of the diagonal $BD$ of the parallelogram $ABCD$ is 18cm. If $P$ and $Q$ are the centroids $\triangle ABC$ and $\triangle ADC$ respectively, length of $PQ$ is,

1. 4cm
2. 12cm
3. 6cm
4. 9cm

Problem 4.

In rhombus $ABCD$, a straight line through $C$ cuts extended $AD$ at $P$ and extended $AB$ at $Q$. If $DP =\displaystyle\frac{1}{2}AB$ the ratio of the lengths of $BQ$ and $AB$ is,

1. 1 : 2
2. 2 : 1
3. 3 : 1
4. 1 : 1

Problem 5.

$A$, $B$ and $C$ are three points on the circumference of a circle. If $AB = AC = 5\sqrt{2}$cm and $\angle BAC=90^0$ the length of radius is,

1. 15cm
2. 5cm
3. 10cm
4. 20cm

Problem 6.

If $PN$ is the perpendicular from a point $P$ on the circumference of a circle of radius 7cm to its diameter $AB$ and the length of the chord $PB$ is 12cm, the length of $BN$ is,

1. $6\displaystyle\frac{5}{7}$ cm
2. $3\displaystyle\frac{5}{7}$ cm
3. $12\displaystyle\frac{2}{7}$ cm
4. $10\displaystyle\frac{2}{7}$ cm

Problem 7.

If the angle subtended by a chord at its center is $60^0$, the ratio between the lengths of the chord and the radius is,

1. $1 : 1$
2. $2 : 1$
3. $\sqrt{2} : 1$
4. $1 : 2$

Problem 8.

$AB$ and $CD$ are two parallel chords of respective lengths 8cm and 6cm on the same side of the center of a circle. The distance between them is 1cm. Then the radius of the circle is,

1. 4cm
2. 5cm
3. 3cm
4. 2cm

Problem 9.

$ABCD$ is a trapezium whose side $AD$ is parallel to $BC$. Diagonals $AC$ and $BD$ intersect at $O$. If $AO = 3$, $CO = x - 3$, $BO = 3x - 19$ and $DO = x - 5$, the value(s) of $x$ will be,

1. 7, 10
2. 7, 6
3. 12, 6
4. 8, 9

Problem 10.

A quadrilateral $ABCD$ circumscribes a circle and $AB=6$cm, $CD=5$cm and $AD=7$cm. The length of side $BC$ is,

1. 3cm
2. 4cm
3. 5cm
4. 6cm

Answers to the exercise problems

Problem 1:  a: $140^0$.

Problem 2: d: 4, 8.

Problem 3: c: 6cm.

Problem 4: b: 2 : 1.

Problem 5: b: 5cm.

Problem 6: d: $10\displaystyle\frac{2}{7}$ cm.

Problem 7: a: 1 : 1.

Problem 8: b: 5cm.

Problem 9: d: 8, 9.

Problem 10: b: 4cm.

---

The detailed solutions to these questions are available in SSC CGL level Solution Set 20 on Geometry 2.

---

Guided help on Geometry in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on Geometry in Suresolv, follow the guide,

Suresolv Geometry Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II and Other Competitive exams.

The guide list of articles includes ALL articles on Geometry and relevant topics in Suresolv and is up-to-date.

---

←   →