
Solve liar and truth-teller riddle second version by common sense reasoning and method
In Liar, Truth-teller, Random-Answerer Riddle, a traveler can ask two questions to choose the safe path out of two. What to ask?
Story of the Liar, Truth-teller and Random answerer Riddle
Months passed since the traveler escaped death by asking the right question to one of the liar and truth-teller who were ready to help.
Now again, the traveler came upon a deep forest where the path divided in two and went into the depths of the jungle.
From the wise man, he already knew about this tough challenge. The wise man told him, “You will again face only two paths going into a deep jungle. One path will lead to the warmth of a friendly village, but the other will lead you to a village of cannibals. They will eat you in no time.”
He continued, “This time when you are at the fork trying to choose the safe path, three men will suddenly appear ready to help. Beware, one of them will be a habitual liar. His answer to any question must always be a lie, a second one will just be the reverse. He always will tell you the truth. But the third one will be the most unpredictable. He will answer to your question absolutely randomly.”
He finished, “Don’t forget, you can ask only two questions to any two of the three one by one to find the safe path. They will understand your question and know which path is safe. They will know the nature of answering of each other as well, but will answer only with YES or NO.”
Recommended time for you to find the safe path: 45 minutes.
Comments
This is a more difficult second version of the classic liar and truth-teller logic puzzle. Logicians who juggle with pure logic by choice won’t find it difficult to ask the right questions.
But we are not logicians. We are common folks who use common sense logic and deductive reasoning in our own way.
Add to that our earlier experience of solving a simpler version of the puzzle. That will surely help, though not necessarily needed.
If you are not a logician, have a go. The experience will be exciting.
You will get better results if you imagine yourself as the traveler.
Solution to liar, truth-teller, random answerer riddle: Stage 1: Analyze end state
Without bothering about any other details, you’ll focus on the two-part end point when you ask the second question,
Part 1: What must you know after the first question?
Part 2: What can be the second and last question you’ll ask?
This is a natural decision anyone should make. It follows the powerful but natural problem solving technique of End state analysis approach.
It should be easier to analyze this end situation to find how the traveler discovers the right path because you will already have useful information gathered by the first question with only one question left.
Solution to liar, truth-teller, random answerer riddle: Stage 2: Specifying requirements for the second question
The chief problem maker is the random answerer as you can’t take any decision based on his answer.
The obvious first conclusion is,
Conclusion 1: You must screen out one of the three helpers by your first question.
You will ask the second question to one of two helpers, not three.
The two selected helpers to ask the second question must be the liar and the truth-teller. At the final stage, if you ask the last question to the random answerer, you won’t be sure of the safe path.
The random answerer must be screened out by the first question.
When determining necessary requirements to solve a problem by end state analysis, never think of HOW and concentrate only on WHAT are the requirements at the End state,
Here, don’t think of how you will screen out the random answerer, but assume you have done it already by your first question.
Solution to liar, truth-teller, random answerer riddle: Stage 3: Forming effective second question
So you have isolated the liar and the truth-teller to ask the second question. Reality is virtual, but it sure is a promising situation,
The original puzzle is broken up into two parts and you are now solving the second part, which is a much simpler puzzle.
This is problem breakdown technique in action.
You know one helper will answer truthfully to your question, and the other will reverse the truth and lie. No uncertainty of the random answerer any more at this imagined final stage.
As a trial, ask a simple question to any of the two,
Trial question 1: “Is the path on my left the safe path?”
As answers from both have to be analyzed for decision (you don’t know who is what), you will realize the unpleasant truth,
Conclusion 2: Answers to the simple question from the two will be reverse to each other.
You won’t be sure of the safe path.
Try out any question of this form and it won’t lead you to solution.
With the awareness of the limitation of this form of question, the critical question you face,
Critical Question 1: What is so special about this type of question?
Now you are not thinking about the CONTENT OF THE QUESTION, but concentrating on the FORM OF THE QUESTION, simply because all questions of this form have failed.
This is the point where you have to jump across an unknown gap and explore what can be other forms of a question.
You may realize in a flash that the word SIMPLE is the KEY. The key pattern of the question is,
Conclusion 3: It is a single and simple question.
And this form won’t work.
What is to be done?
Conclusion 4: Ask a COMPOUND QUESTION comprising MORE THAN ONE QUESTION.
This is applying Problem solving technique of Changing the property of the main entity.
The main entity is the Question and its Property of number of component questions is changed from 1 to 2.
By the rules of English language, you can join many single sentences together to form a compound sentence.
Now your attention shifts to the ANSWER itself.
Solution to liar, truth-teller, random answerer riddle: Stage 4: Specification of second question
This is a very natural way to solve problems—to first analyze and understand characteristics of the end result, all the while comparing with given information.
Thinking ahead in this direction, raise the most important QUESTION at this point,
Critical Question 2: What must be the NATURE OF THE ANSWER from the two so that you would be sure of the safe path?
You already know that the answer to a simple question from one helper will be NO and the other, YES, just the reverse. So you can make a firm conclusion,
Conclusion 5: If the answers from the two are reverse to each other, you won’t find the safe path. Answer from both must be same.
This is a revelation to you and in problem solving terminology, discovery of the key pattern. You have now a precise requirement of their answers.
Naturally, the answer will be YES or NO. But if it is NO, both will answer NO. The same must also be true for YES for knowing safe path.
This is an important breakthrough.
Combining two results, specify the second question,
- The question must be a compound question comprising more than one question, and
- Answer to the second question must be same if asked to each of the two helpers selected by the first question.
Solution to liar, truth-teller, random answerer riddle: Stage 5: Finalize the second question
With clear idea of the nature of the answer and form of the question, a possible safe question to ask would be,
Trial question 2: “Are you the truth-teller and do you think the path to my left is the safe path?”
This is a simple, conventional way to combine two questions.
Possible situations are,
Situation 1: The helper asked is the truth-teller and the left path is the safe path: Final answer: YES.
Situation 2: The helper asked is the liar and the left path is the safe path. Correct answer to first part will be NO, so that answer to the combined question will be NO. Habitual liar will reverse this NO to YES. Final answer will be YES.
So if the answer to your question is YES, you might think that the left path is the safe path.
Before being fully confident, let us evaluate the other two possibilities,
Situation 3: The helper asked is the truth-teller and the left path is NOT the safe path: Final answer: NO.
Situation 4: The helper asked is the liar and the left path is NOT the safe path, answer to both the parts are NO that will be reversed by the liar to YES. Final answer: YES.
This answer is reverse to the answer of other helper and violates the requirement specification of same answer by both.
Just on the brink of success, you find that joining of two questions simply by ‘and’ won’t work.
You have chosen an easy way to combine the two questions without thinking much on how to combine. You need to form the right way to combine the two parts of the last question.
You decide to analyze the results of the trial to get clues on how to combine.
Question: Why and where did combining two questions by ‘and’ fail?
As you concentrate on analyzing the result of your last try to answer this question, you realize,
- Answers to the two component question 1 and question 2 are independent of each other, and
- No need to think about the answer of the truth-teller. Your goal is to force the liar to reverse the answer of the truth-teller twice to match his final answer.
In the Situation 2, using ‘and’ for joining, you could indeed force the liar to reverse the answer of the truth-teller twice.
But in the Situation 4, where correct answer to both part questions were NO, the liar would first combine the two to a final answer of NO and then by habit would reverse it to YES.
To force the liar to reverse true answer twice by two questions with certainty then,
One question must be DEPENDENT on the other so that in both possible scenarios for the liar, he would be forced to reverse the truth twice.
The successful method of combining the two questions must make sure that,
The liar would reverse correct answer to the INDEPENDENT first question. Thinking that he has answered it true to his habit, he would face the second DEPENDENT question. While answering the second dependent question, he would again reverse answer to the first independent question in line with his nature. The correct answer will be reversed twice to become correct ultimately.
What is the other method of joining two questions that will achieve this result?
Again, your common experience of using the language helps you for the final breakthrough.
There must be only one real question but asked twice in the commonly used form,
What will be your answer if I ask you whether the QUESTION is true?
By the rules of language, the liar must answer first the component question,
Whether the QUESTION is true.
The liar reverses the correct answer to this first component (and independent) question and forms the INTERMEDIATE RESULT in line with his nature of answering. With this knowledge, he faces the second part question,
What will be your answer if INTERMEDIATE RESULT is true?
Now he will have no choice other than to reverse the intermediate result which according to him is correct and form the final result. Final result becomes the reverse of the intermediate result.
As INTERMEDIATE RESULT has itself been reversed once from correct result, the final result returns to the value of the correct result by this double reversal.
Following is the schematic of this mechanism,
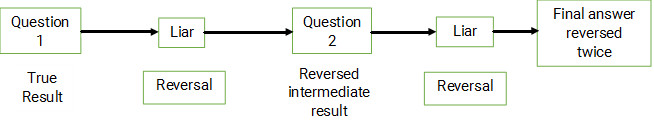
With confidence, you will now form the single question that will lead you to the safe path,
Second question solution: What will be your answer if I ask you whether the path to my left is the safe path?
You will ask this second question to any of the two helpers. If the answer is YES, you take the left path, and if NO, you take the right path.
There cannot be any other possibility—you have indeed forced the liar to reverse the true answer twice to match the final answer of the truth-teller in both the situations.
This is though in the future. You need to first drop the random answerer by the first question.
So you take up the second challenge of SCREENING OUT THE RANDOM ANSWERER BY YOUR FIRST QUESTION.
Do you realize you are going from End to Start, or back to front? This is the well-known general problem solving technique of Working backwards approach.
Solution to liar, truth-teller, random answerer riddle: Stage 6: Screening out random answerer
Nature of second question is now known. It will be on the choice of path.
As all three know about each other’s nature of answering, the conclusion is easy to make,
Conclusion 6: The first question must be about how the helpers answer.
This is an easy conclusion to make, but what should be the first question to ask? That is not easy to decide.
So you take up the third mental trial on the question. Pointing to one of the helpers, you ask another,
Trial question 3: “Do you think he is the habitual liar?”
Assume the questioned helper is the truth-teller and pointed helper is the liar. Answer will be YES. But if the pointed helper is the random answerer, answer will be NO. Without knowing the nature of the questioned helper, your confusion will increase.
But why the confusion is so much with the trial question of this form?
This is because the approach is too simple and,
Combination of possible outcomes are too many as you have covered only two of the three helpers by the question on the nature of only one other helper, not both.
A general principle of questioning
When you have a single question to ask, you must form the question that will give you maximum information.
Realize that this approach is the most effective when you have only one arrow in your quiver of arrows. The arrow must be the most powerful arrow.
The critical question you raise to yourself,
Critical Question 3: What is the question that will give you maximum information about the nature of answering of the helpers?
As you think of possible answers to the leading question, you realize you face again a gap you must jump across.
Common knowledge about getting maximum information on things by a single question: COMPARE NATURE OF THINGS
As you have to ask the first question to any of the three,
Conclusion 7: To cover maximum number of helpers, include both the other two in your question, and
To get maximum information, you will ask the question comparing the nature of answering of the other two.
You will form a trial question again that you ask to a FIRST HELPER,
Trial question 4: “Is the THIRD HELPER (point out) more truthful than the SECOND HELPER (point out)?”
As the trial question is indeed the most promising one, fulfilling all requirements that you are aware of, you will take the trouble to compile ALL possible answer scenarios in the following table.
>

When you scan through the possible answers in the six different combination scenarios, you realize
Conclusion 8: For the final question, if you choose the SECOND HELPER for answer YES, you will get rid of the Random answerer for the first and third scenarios.
Likewise, conclude,
Conclusion 9: For the final question, if you choose the THIRD HELPER when answer is NO you will avoid the random answerer for the second and fourth scenarios.
But what about the last two scenarios?
Simple.
Logic and Reason: As you are choosing the second helper for answer YES and the third helper for answer NO, the first helper questioned random answerer is automatically dropped for the last two scenarios (in no case you are choosing the first helper).
Desired result is achieved by asking the first question to any First helper, “Is the THIRD HELPER (point out) more truthful than the SECOND HELPER (point out)?”
You will ask now the second question to any of the two helpers selected,
Second question solution: What will be your answer if I ask you whether the path to my left is the safe path?
If the answer is YES, you will take the left path, and if it is NO, you take the right path.
And again, you will be safe.
The problem solving techniques, concepts and common knowledge used
- End state analysis approach: Analyzing the desired result or last action first. Goal is to gain more knowledge about the last action for achieving the desired result.
- Problem breakdown technique: This a natural problem solving technique of splitting a large problem into smaller manageable problem chunks to solve part by part and finally combine the results of the individual parts.
- Refining requirement specification in steps: Knowing precise requirements of the answer first and the question second simplified the steps to the solution greatly.
- Question, analysis and answer or QAA technique: The problem is simplified stage by stage by asking a series of relevant questions, analyzing problem details for getting the answer and actually forming the answer.
- Property change analysis technique: Exploring how many ways the key property of a key entity can be changed and assessing promise of each change. It often proves to be crucial in solving a complex problem. Great innovation can be created by this technique.
- Elementary knowledge in language: Basic domain concepts: for combining two component questions to a single compound question, but in different ways.
- Well-formed trials or experiments: To learn more of the problem.
- Working backwards approach: A powerful problem solving technique that moves from end to start.
- Step by step deductive reasoning: By using all the above and discovering key patterns of information for solving the problem with complete confidence.
End note
Our focus all through was to think as the traveler in a simple way and find the safe path using systematic reasoning and problem solving techniques drawn out of common knowledge and experience step by step.
Do you want to know how to solve difficult problems easily without wasting time on random attempts?
Our new ebook on puzzle solutions by innovative methods will show you just that.
Puzzles for Adults: 50 Brain Teasers with Step-by-Step Solutions: Boost Your Power of Problem Solving
You will enjoy solving its 50 challenging brain teasers and at the same time boost your power of problem solving from the innovative step by step solutions rich with new ideas.
The general problem solving techniques are collected first time in a separate chapter.
It's not just solving puzzles. It is also learning innovative problem solving techniques that will change the way you try to solve ANY problem.
You may buy the eBook at Amazon or at Google Play.
Puzzles you may enjoy
Easy to hard brain teasers with systematic solutions
Challenging brain teasers with solutions: Long list.
This will always be the most up-to-date full list with the brain teasers classified into categories that can be browsed separately.
You may also click on the category term link below to enjoy the brain teasers that are classified in the present category.
For example, if the category term link shown below is "Riddle", click on it to go through all the Riddles.
