Trigonometry questions with solutions: SSC CGL Tier 2 Set 12
Trigonometry Questions with Solutions for SSC CGL Tier 2 Set 12 explains how 10 difficult trigonometry questions can be solved in 12 minutes' time.
For best results take the test first at,
12th SSC CGL Tier II level question set and 3rd on Trigonometry.
Solutions to 10 Selected Trigonometry Questions for SSC CGL Tier 2 Set 12 - testing time was 12 mins
Problem 1.
If $2abcos \theta + (a^2-b^2)sin \theta=a^2+b^2$ then the value of $tan \theta$ is,
- $\displaystyle\frac{1}{2ab}(a^2+b^2)$
- $\displaystyle\frac{1}{2}(a^2-b^2)$
- $\displaystyle\frac{1}{2}(a^2+b^2)$
- $\displaystyle\frac{1}{2ab}(a^2-b^2)$
Solution 1. Problem analysis: identification of promising path
We look at the target $tan \theta$ and chalk out a possible solution path—if we find out $sec \theta - tan \theta$, we should be able to find $sec \theta + tan \theta$ also by just reversing the value. With these two values in the pocket, finding $tan \theta$ won't pose any problem. There is rarely an easier path to find $tan \theta$.
This decision is based on the rich concept of friendly trigonometric function pairs. and the presence of $sin \theta$ and $cos \theta$ in the given expression.
Rich Concept of friendly trigonometric function pairs
Let us explain this with the first example pair of functions,
$sec\theta$ and $tan\theta$.
We have,
$sec\theta + tan\theta = \displaystyle\frac{(sec\theta +tan\theta)(sec\theta - tan\theta)}{sec\theta - tan\theta}$
$\hspace{25mm}=\displaystyle\frac{1}{sec\theta -tan\theta}$, because $sec^2\theta - tan^2\theta=1$.
The result is somewhat similar to surd rationalization.
In the same way, in the case of the second friendly function pair of $cosec\theta$ and $cot\theta$, we get,
$cosec\theta + cot\theta =\displaystyle\frac{1}{cosec\theta - cot\theta}$.
The inherent friendship mechanism of the third friendly function pair, $sin\theta$ and $cos\theta$ is well known and is used very frequently,
$sin^2 \theta + cos^2 \theta=1$.
Most promising course of action from Initial problem analysis
Objective is to transform the expression in $sec \theta$ and $tan \theta$. This is what we call promising course of action. We may not be absoluterly sure of all the steps to the final solution, but we choose the most promising course of action at a critical point in the solution process. This promise we know from our Initial problem analysis and domain knowledge.
Usually the most promising course action straightaway leads to the solution, at least in the case of Competitive math problem solving.
In real life complex problems, we take up the most promising course of action and evaluate results to tune the action further.
We are solving again..
But how to get $sec \theta + tan \theta$ or $\sec \theta -tan\theta$ from the given expression!
The first step with this penultimate goal in mind would surely be converting the $sin \theta$ and $cos \theta$ to $tan \theta$ and $sec \theta$.
Solution 1. Problem solving execution
The given equation,
$2abcos \theta + (a^2-b^2)sin \theta=a^2+b^2$,
Dividing by $cos \theta$ and collecting terms with common $a^2$ and $b^2$,
$2ab=a^2(sec \theta - tan \theta) + b^2(sec \theta + tan \theta)$.
Dividing by $ab$ to increase harmony in the expression further,
$\displaystyle\frac{a}{b}(sec \theta -tan \theta)+\displaystyle\frac{b}{a}\times{\displaystyle\frac{1}{sec \theta - tan \theta}}=2$.
This is familiar ground of algebraic simplification, but greatly aided by the most potent trigonometric relation,
$sec \theta + tan \theta=\displaystyle\frac{1}{sec \theta - tan \theta}$.
We will now simplify the equation to an one variable equation by Substituting $x$ in place of the repeating expression $\displaystyle\frac{a}{b}(sec \theta - tan \theta)$. This is a powerful algebraic problem solving technique in reducing the complexity of the expression being simplified. This also results in reduction of number of variables as well as number of terms.
The last stage is in the form of inverses,
$x + \displaystyle\frac{1}{x}=2$, where, $x=\displaystyle\frac{a}{b}(sec \theta - tan \theta)$,
This is a quadratic equation in $x$,
$x^2 - 2x + 1=(x-1)^2=0$.
So,
$x=\displaystyle\frac{a}{b}(sec \theta - tan \theta) = 1$.
As we have thought at the very beginning, we have obtained the value of $sec \theta - tan \theta=\displaystyle\frac{b}{a}$,
So,
$sec \theta + tan \theta=\displaystyle\frac{a}{b}$.
Subtracting the first from the second and dividing by 2,
Or, $tan \theta =\displaystyle\frac{1}{2ab}(a^2-b^2)$.
Answer: Option d: $\displaystyle\frac{1}{2ab}(a^2-b^2)$.
Key concepts and techniques used: End state analysis approach -- Use of most potent friendly trigonometric function pair -- Working backwards approach -- Initial possible approach -- Most promising course of action -- Initial problem analysis -- basic trigonometry concepts -- Input transformation to more promising form of relation -- Algebraic simplification techniques -- Substitution technique -- Variable reduction technique -- roots of a quadratic equation -- Analytical approach example.
Though the problem looked difficult, most of the solution steps could be done in mind.
This is a good example of analytical approach to problem solving.
Problem 2.
$\displaystyle\frac{\sin^2 \theta}{\cos^2 \theta}+\displaystyle\frac{\cos^2 \theta}{\sin^2 \theta}$ is equal to,
- $\displaystyle\frac{1}{\sin^2 {\theta}\cos^2 \theta}$
- $\displaystyle\frac{1}{\sin^2 {\theta}\cos^2 \theta} -2$
- $\displaystyle\frac{1}{\tan^2 \theta - \cot^2 \theta}$
- $\displaystyle\frac{\sin^2 \theta}{\cot \theta - \sec \theta}$
Solution 2. Problem solving execution
As direct addition would result in $sin^4 \theta + cos^4 \theta$ in the numerator, leaving this uncomfortable path, we would transform to $tan^2 \theta$ and $cot^2 \theta$ and break up each term further in terms of $sec^2 \theta$ and $cosec^2 \theta$,
$\displaystyle\frac{\sin^2 \theta}{\cos^2 \theta}+\displaystyle\frac{\cos^2 \theta}{\sin^2 \theta}$
$=tan^2 \theta + cot^2 \theta$
$=sec^2 \theta + cosec^2 \theta - 2$
$=\displaystyle\frac{1}{\sin^2 {\theta}\cos^2 \theta} -2$, as $sin^2 \theta + cos^2 \theta=1$ in the numerator after taking inverse and then adding the two terms.
Answer: Option b: $\displaystyle\frac{1}{\sin^2 \theta.cos^2 \theta} -2$.
We have first got rid of fraction terms. effectively reducing number of terms from 4 to 2 considering the 2 numerators and denominators. Transforming again using more potent relations $sec^2 \theta -1= tan^2 \theta$ and $cosec^2 \theta-1=cot^2 \theta$, we reach very near to solution.
Key concepts and techniques used: Useful pattern identification and exploitation -- basic trigonometry concepts -- friendly trigonometric function pairs concept -- input transformation technique -- efficient simplification.
All steps could easily be done in mind.
Problem 3.
If $cos \theta + sec \theta = 2$, then the value of $cos^5 \theta + sec^5 \theta$ is,
- $-2$
- $2$
- $1$
- $-1$
Solution 3. Problem analysis
Noticing the inverse relation of the form, $x + \displaystyle\frac{1}{x}=2$ and the target expression also in higher power of inverses, for a moment we considered using the algebraic path of evaluating inverses.
Then noticing the given expression closely we detected the possibility for finding the value of $cos \theta$ directly.
Solution 3. Problem solving execution
Given expression,
$cos \theta + sec \theta = 2$,
Or, $cos^2 \theta - 2cos \theta + 1=0$
Or, $(cos \theta - 1)^2=0$
Or, $cos \theta = sec \theta =1$
So,
$cos^5 \theta + sec^5 \theta=2$
Answer: Option b: $2$.
Key concepts and techniques used: Pattern identification technique -- End state analysis approach -- Most promising course of action -- Basic algebraic concepts.
All the processing could easily be done mind. The main task was to identify the useful pattern.
Problem 4.
$sin(\alpha + \beta -\gamma)=cos(\beta + \gamma -\alpha)=\displaystyle\frac{1}{2}$ and $tan(\gamma + \alpha -\beta)=1$. If $\alpha$, $\beta$ and $\gamma$ are positive acute angles, value of $2\alpha + \beta$ is,
- $105^0$
- $110^0$
- $115^0$
- $120^0$
Solution 4. Problem analysis
From acute angle conditions we would get actual values of the three angle expressions within brackets. With three variables and three linear equations it is always possible to solve for any expression in these three angles.
Solution 4. Problem solving execution
First relation,
$sin(\alpha + \beta -\gamma)=\displaystyle\frac{1}{2}$
So,
$\alpha + \beta -\gamma=30^0$.
Second relation,
$cos(\beta + \gamma -\alpha)=\displaystyle\frac{1}{2}$,
So,
$\beta + \gamma -\alpha=60^0$.
The third relation,
$tan(\gamma + \alpha -\beta)=1$,
So,
$\gamma + \alpha -\beta=45^0$.
Adding the first two equations and dividing by 2 we get,
$\beta=45^0$, and adding the first and third equations we get,
$2\alpha=75^0$.
So,
$2\alpha + \beta=120^0$
Answer: Option d: $120^0$.
Key concepts and techniques used: Initial problem analysis -- Trigonometric ratio values -- linear algebraic equations -- Analytical approach example.
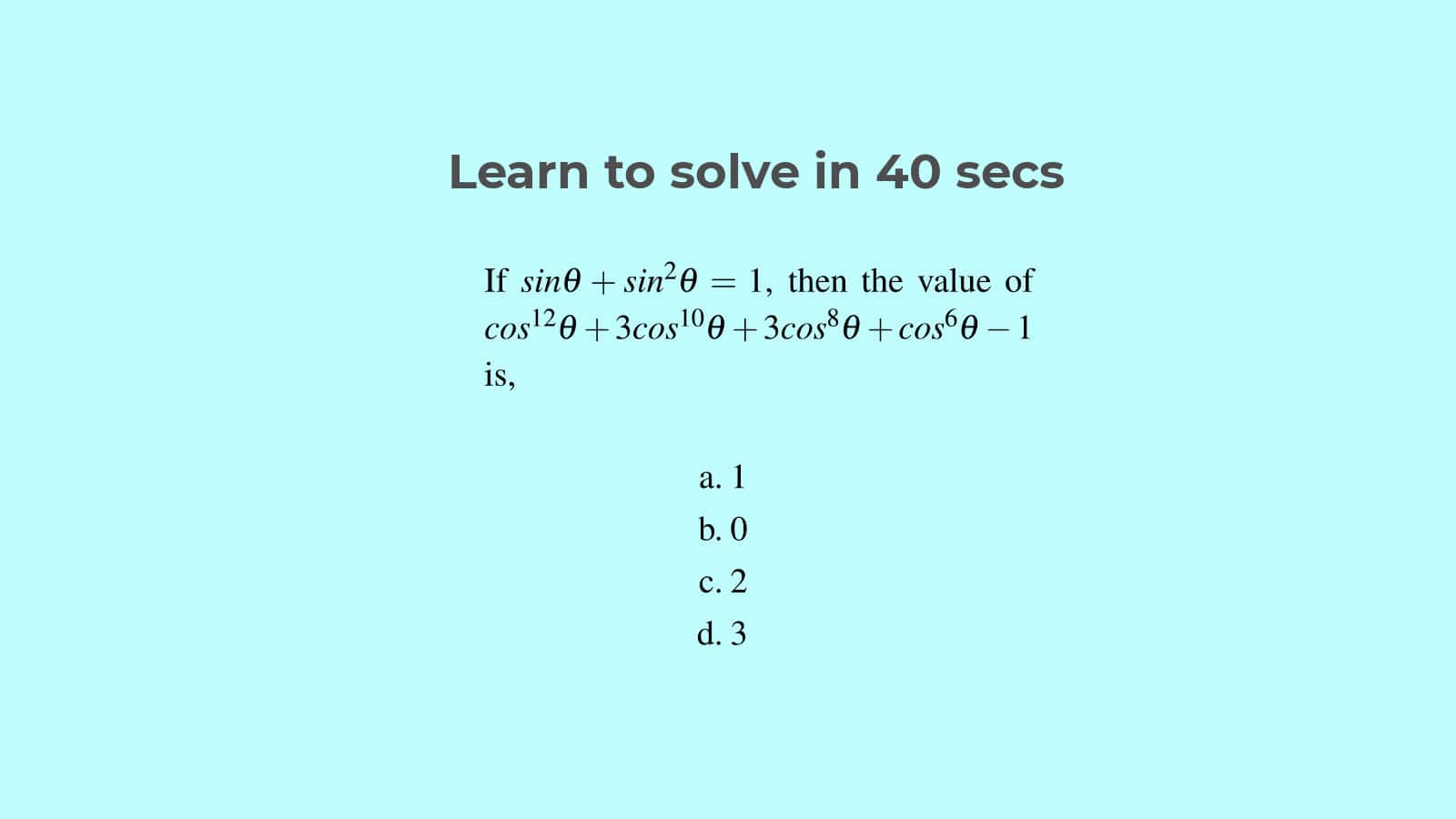
Problem 5.
If $sin \theta + sin^2 \theta=1$, then the value of $cos^{12} \theta + 3cos^{10} \theta + 3cos^{8} \theta + cos^6 \theta - 1$ is,
- $1$
- $0$
- $2$
- $3$
Solution 5. Problem analysis and input transformation
As the target expression is more complex, it is apparent that we have to use the input expression to simplify it. But in the present form no apparent promising course of action could be found. The three terms caused the basic problem. If only we could have two terms in the equation, we might be able to replace one term by the other in the target expression.
On closer inspection the input equation could be transformed in the desired manner,
$sin \theta + sin^2 \theta =1$,
Or, $sin \theta = 1 - sin^2 \theta=cos^2 \theta$.
Solution 5. Problem solving execution
Now the task is cut out. We need to express the target expression in terms of $cos^2 \theta$.
The two coefficients of value 3 could give the help to convert $cos$ expression to a cube of sum,
$E=cos^{12} \theta + 3cos^{10} \theta + 3cos^{8} \theta + cos^6 \theta - 1$
$=(cos^4 \theta + cos^2 \theta)^3 - 1$
$=(sin^2 \theta+cos^2 \theta)^3-1$
$=0$
Answer: Option b: $0$.
Key concepts and techniques used: Input transformation -- Term reduction technique -- Useful pattern identification -- Substitution -- Friendly trigonometric function pair -- Analytical approach example.
Problem 6.
The value of $sec \theta\left(\displaystyle\frac{1+sin \theta}{cos \theta}+\displaystyle\frac{cos \theta}{1+sin \theta}\right) - 2tan^2 \theta$ is,
- 4
- 0
- 2
- 1
Solution 6. Problem analysis
We mark the symmetry and possibility of transforming the expression in terms of sum of $sec \theta$ and $tan \theta$. The sum and subtraction expressions of $sec$ and $tan$ (and also $cosec$ and $cot$) we call golden trigonometric function pairs because of their great potential for simplification.
Solution 6. Problem solving execution
The given expression is,
$E=sec \theta\left(\displaystyle\frac{1+sin \theta}{cos \theta}+\displaystyle\frac{cos \theta}{1+sin \theta}\right) - 2tan^2 \theta$.
Multiply the first and the second terms in the brackets, both numerator and denominator, by $sec \theta$,
$E=sec \theta\left(sec \theta+tan \theta+\displaystyle\frac{1}{sec \theta+tan \theta}\right) - 2tan^2 \theta$
Now substitute inverse of $\displaystyle\frac{1}{sec \theta + tan \theta}=sec \theta - tan \theta$ to the second term and simplify,
$E=sec \theta.2sec \theta - 2tan^2 \theta$
$=2(sec^2 \theta - tan ^2 \theta)$
$=2$
Answer: Option c: 2.
Quick, elegant and all in mind.
Key concepts and techniques used: Basic trigonometry copncepts -- Trigonometric function transformation -- friendly trigonometric function pairs.
Problem 7.
If $4cos^2 \theta - 4\sqrt{3}cos \theta + 3=0$ and $0^0 \leq \theta \leq 90^0$, then the value of $\theta$ is,
- $60^0$
- $90^0$
- $30^0$
- $45^0$
Solution 7. Problem analysis and solving execution
In this problem everything hinges on finding the exact value of $\theta$ directly from the quadratic equation in $cos \theta$.
On closer inspection we could indeed express the given expression as a square of sum,
Given expression,
$4cos^2 \theta - 4\sqrt{3}cos \theta + 3=0$,
Or, $(2cos \theta - \sqrt{3})^2=0$,
Or, $cos \theta =\displaystyle\frac{\sqrt{3}}{2}$.
So,
$\theta=30^0$.
Answer: Option c: $30^0$.
Key concepts used: Pattern identification technique -- input transformation -- roots of qudratic equation -- Basic trigonometry concepts.
Problem 8.
If $sin \theta + cos \theta=\sqrt{2}sin(90^0 - \theta)$ then the value of $cot \theta$ is,
- $\sqrt{2}-1$
- $\sqrt{2}+1$
- $-\sqrt{2}+1$
- $-\sqrt{2}-1$
Solution 8. Problem solving execution
We will use first complementary trigonometric function concept, $sin (90^0 - \theta)=cos \theta$,
Given expression,
$sin \theta + cos \theta=\sqrt{2}sin(90^0 - \theta)$,
Or, $sin \theta + cos \theta=\sqrt{2}cos \theta$,
Dividing throughout by $cos \theta$,
Or, $tan \theta + 1=\sqrt{2}$,
Or, $tan \theta=\sqrt{2}-1$,
Or, $cot \theta=\displaystyle\frac{1}{\sqrt{2}-1}$,
Or, $cot \theta = \sqrt{2}+ 1$, by surd rationalization, multipltying both numerator and denominator by $\sqrt{2}+1$.
Answer: Option b: $\sqrt{2}+1$.
Key concepts and techniques used: Basic trigonometry concepts -- complementary angle concepts -- Surd rationalization.
Problem 9.
If $x=asin \theta-bcos \theta$ and $y=acos \theta + bsin \theta$, then which of the following is true?
- $x^2+y^2=a^2+b^2$
- $\displaystyle\frac{x^2}{a^2}+ \displaystyle\frac{y^2}{b^2}=1$
- $x^2+y^2=a^2-b^2$
- $\displaystyle\frac{x^2}{y^2}+ \displaystyle\frac{a^2}{b^2}=1$
Solution 9. Problem analysis
As all the choice values have squares, we will first form the squares of $x$ and $y$.
Solution 9. Problem solving execution
Given equations,
$x=asin \theta-bcos \theta$
Squaring,
$x^2=a^2sin^2 \theta - 2absin \theta.cos \theta + b^2cos^2 \theta$,
Similarly squaring the second equation,
$y^2=a^2cos^2 \theta + 2absin \theta.cos \theta + b^2sin^2 \theta$.
Adding the two and collecting the terms with coefficients $a^2$ and $b^2$,
$x^2 + y^2=a^2 + b^2$, as factor $sin^2 \theta+ cos^2 \theta=1$
Answer: Option a: $x^2 + y^2=a^2 + b^2$.
Key concepts and techniques used: End state analysis approach -- friendly trigonometric function pairs -- target driven simplification.
All in mind and in quick time.
Problem 10.
If $tan \theta=\displaystyle\frac{a}{b}$, then the value of $\displaystyle\frac{asin^3 \theta - bcos^3 \theta}{asin^3 \theta + bcos^3 \theta}$ is,
- $\displaystyle\frac{a^4-b^4}{a^4+b^4}$
- $\displaystyle\frac{a^3+b^3}{a^3-b^3}$
- $\displaystyle\frac{a^3-b^3}{a^3+b^3}$
- $\displaystyle\frac{a^4+b^4}{a^4-b^4}$
Solution 10. Problem analysis
The target being in the form perfectly suitable for applying Componendo dividendo technique $\left(\text{in the form }\displaystyle\frac{x-y}{x+y}\right)$, the very first step we would take is to apply the technique on the target expression. After the result is simplified we would substitute the value of $tan \theta$ and again reapply the Componendo dividendo technique to get the target value.
The method is clear and once we show it, you will easily understand the mechanism. But yes, you have to know componendo dividendo technique.
Rich algebraic technique of Componendo and dividendo
Problem: Simplify, $\displaystyle\frac{x - y}{x + y} = \displaystyle\frac{1}{3}$.
First we add 1 to both sides of the equation,
$1+\displaystyle\frac{x - y}{x + y} = 1+\displaystyle\frac{1}{3}$,
Or, $\displaystyle\frac{2x}{x+ y} = \displaystyle\frac{4}{3}$.
Next we subtract both sides of the equation from 1,
$1-\displaystyle\frac{x - y}{x + y} = 1-\displaystyle\frac{1}{3}$,
Or, $\displaystyle\frac{2y}{x + y} = \displaystyle\frac{2}{3}$.
Now we divide the first result by the second,
$\displaystyle\frac{x}{y} = 2$, a greatly simplified expression with two terms in the numerator and denominator reduced to single terms.
This is a powerful algebraic technique frequently applied whenever we encounter the special form of given expression.
Solution 10. Problem solving execution
Let target expression,
$\displaystyle\frac{asin^3 \theta - bcos^3 \theta}{asin^3 \theta + bcos^3 \theta}=x$.
Applying componendo dividendo technique,
$\displaystyle\frac{1+x}{1-x}=\frac{asin^3 \theta}{bcos^3 \theta}=\displaystyle\frac{a}{b}tan^3 \theta$
$=\displaystyle\frac{a^4}{b^4}$.
Reapplying the componendo dividendo technique (this time first subtract 1 from the equation to get numerator $2x$, next add 1 to the equation to get numerator $2$ and then take ratio),
$x=\displaystyle\frac{a^4-b^4}{a^4+b^4}$.
Answer: Option a: $\displaystyle\frac{a^4-b^4}{a^4+b^4}$.
Key concepts and techniques used: Useful pattern recognition -- basic trigonometry concepts -- componendo dividendo technique -- Analytical approach example.
Note: You will observe that in many of the Trigonometric problems basic and rich algebraic concepts and techniques are to be used. In fact that is the norm. Algebraic concepts are frequently used for quick solutions of Trigonometric problems.
Guided help on Trigonometry in Suresolv
To get the best results out of the extensive range of articles of tutorials, questions and solutions on Trigonometry in Suresolv, follow the guide,
The guide list of articles is up-to-date.