SSC CGL Tier II level Solution Set 6, Geometry 3

6th SSC CGL Tier II Solution Set on Geometry

SSC CGL Geometry Solution Set 6 for Tier II gives you clear idea on how to solve the 10 questions in SSC CGL Tier II Geometry question set 6 in 12 mins.

If you have not yet taken this test you should take it by referring to the SSC CGL Tier II level question set 6 on Geometry 3 before going through the solutions.

---

Solutions to SSC CGL Geometry questions set 6 for Tier II - answering time was 12 mins

Problem 1.

Lengths of three perpendiculars from a point inside an equilateral triangle on the three sides being 16 cm, 25 cm and 28 cm, the area of the triangle (in cm$^2$) will be.

1. $1587\sqrt{3}$
2. $1587$
3. $2116$
4. $2116\sqrt{3}$

Solution 1 - Problem analysis

The following figure describes the problem solution,

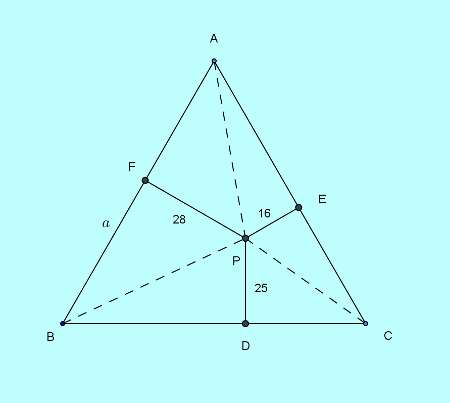
It is observed that the point P divides the area of the triangle into three triangles with bases as the main triangle sides, say of length $a$, and heights of given lengths. Thus we get a linear relation of area of triangle in terms of triangle side length $a$.

As area of an equilateral triangle is, $\displaystyle\frac{\sqrt{3}}{4}a^2$, equating the two relations we get the value of $a$ and then area of the triangle.

Solution 1 - Problem solving execution

Proceeding in this way,

$A_{ABC}=A_{PAB}+A_{PBC}+A_{PCA}$,

Or, $A_{ABC}=\displaystyle\frac{1}{2}a(28+25+16)=\displaystyle\frac{69}{2}a$.

Again $\triangle ABC$ being an equilateral triangle of side length $a$ its area is,

$A_{ABC}=\displaystyle\frac{\sqrt{3}}{4}a^2=\displaystyle\frac{69}{2}a$,

Or, $a= \displaystyle\frac{4}{\sqrt{3}}\times{\displaystyle\frac{69}{2}}=46\sqrt{3}$.

So the desired area of the triangle is,

$A_{ABC}=\displaystyle\frac{\sqrt{3}}{4}a^2=\displaystyle\frac{\sqrt{3}}{4}\times{(46\sqrt{3})^2}=1587\sqrt{3}$ cm$^2$.

Answer: a: $1587\sqrt{3}$.

Key concepts used: Area decomposition technique -- key pattern identification -- area of a triangle -- area of an equilateral triangle from its side length -- basic algebraic concepts -- algebraic equation solving.

Problem 2.

If the ratio of sines of angles of a triangle is $1:1:\sqrt{2}$, the ratio of square of the greatest side to sum of the squares of the other two sides is,

1. 1:2
2. 1:1
3. 2:1
4. 3:4

Solution 2 - Problem analysis and solving

The problem definition clearly urges us to use the important concept of laws of Sines in a triangle,

$\displaystyle\frac{a}{\ sin \ A}=\frac{b}{\ sin \ B}=\frac{c}{\ sin \ C}$,

where the sides are, $a$, $b$ and $c$ and the corresponding opposite angles are $\angle A$, $\angle B$ and $\angle C$.

Assuming the angles corresponding to the three ratio values as, $\angle A$, $\angle B$, and $\angle C$ and using the first pair of ratio variables and laws of sines we get,

$\displaystyle\frac{a}{\ sin \ A}=\frac{b}{\ sin \ B}$,

Or, $\displaystyle\frac{a}{b}=\frac{\ sin \ A}{\ sin \ B}=1$.

So,

$a=b$, and

$\angle A=\angle B$.

Using the second pair of ratio value with laws of sines,

$\displaystyle\frac{b}{c}=\frac{\ sin \ B}{\ sin \ C}=\frac{1}{\sqrt{2}}$,

Or, $c=\sqrt{2}b$.

So, $c$ is the largest side.

Ratio of the three sides is,

$a:b:c=b:b:\sqrt{2}b$.

Thus ratio of square of the largest side to the sum of squares of the other two sides is,

$\displaystyle\frac{2b^2}{b^2+b^2}=1:1$

Answer: b: 1:1.

Key concepts used: Laws of sines -- basic ratio concepts -- simplification.

Problem 3.

The perimeters of similar triangles $\triangle ABC$ and $\triangle PQR$ are 24 cm and 36 cm respectively. If $AB=10$ cm, then $PQ$ (in cm) is,

1. 18
2. 12
3. 15
4. 16

Solution 3 - Problem analysis and solving

As the $\triangle ABC$ and $\triangle PQR$ are similar, the ratio of corresponding sides are equal,

$\displaystyle\frac{AB}{PQ}=\frac{BC}{QR}=\frac{CA}{RP}=p$, say.

So perimeters of the two triangles are related as,

$AB+BC+CA=p(PQ+QR+RP)$,

Or, $24=36p$,

Or, $p=\displaystyle\frac{24}{36}=\frac{2}{3}$.

Again,

$AB=10=p\times{PQ}=\displaystyle\frac{2}{3}PQ$.

Finally then,

$PQ=\displaystyle\frac{3}{2}\times{10}=15$ cm

Answer: c: 15.

Key concepts used: Basic ratio concepts -- use of two perimeter values to find the ratio coefficient -- applying the ratio coefficient on given side length to find the corresponding side length of the second triangle -- perimeter concept -- basic algebra concepts.

Problem 4.

In $\triangle ABC$, the bisector of $\angle A$ is AP and it meets BC at P. If a line DE intersects AB, AP and AC at D, Q and E respectively and is perpendicular to AP, then which of the following is true?

1. AQ=QP
2. AD=AE
3. BP=PC
4. QP=EC

Solution 4 - Problem analysis and solving

The following figure depicts the given problem.

Let us analyze the angles in the two triangles, $\triangle AQD$ and $\triangle AQE$.

The triangles are similar as $\angle DAQ=\angle EAQ$ and a second pair of angles are $90^0$. So the third pair of angles are also equal, and the triangles are similar to each other.

As we know, in similar triangles ratio of corresponding sides are equal.

But as, the two triangles have a common side AQ which represents a pair of corresponding sides in the two adjoining triangles, the ratio of corresponding sides are $1:1$. In other words the corresponding sides are equal.

This results in, $AD=AE$.

Answer: b: AD=AE.

Key concepts used: Similar triangle properties -- deductive reasoning -- key pattern identification and use.

Note: The triangles in fact are congruent with all corresponding sides and all corresponding angles equal to each other. AD and AE are two radii of a circle with centre at $O$ bisecting the chord DE.

Problem 5.

In a quadrilateral ABCD, $\angle B=90^0$, and $AD^2=AB^2+BC^2+CD^2$. Then the $\angle ACD$ is,

1. $45^0$
2. $60^0$
3. $50^0$
4. $90^0$

Solution 5 - Problem analysis and solving

The figure depicting the problem is shown below.

Given $\angle ABD=90^0$ is the only angle information. the other relationship is on sides, that too of squares. This immediately urges us to use the Pythagoras theorem that converts the property of a $90^0$ in a triangle to a relationship of squares of sides.

In $\triangle ABC$ formed by the diagonal AC and the two sides AB and BC,

$AC^2=AB^2+BC^2$.

Using this result with the given relation of squares of sides,

$AD^2=AB^2+BC^2+CD^2=AC^2+CD^2$.

It means, in the $\triangle ACD$, AD must be the hypotenuse. In other words, $\angle ACD=90^0$.

Answer: d: $90^0$.

Key concepts used: Visualization -- Pythagoras theorem.

Problem 6.

In figure below the lengths of the four sections of the two diagonals of a trapezium ABCD, namely, AO, BO, CO and DO are given.

<

Find the possible values of $x$.

1. 6, 8
2. 8, 9
3. 9, 7
4. 7, 8

Solution 6 - Problem analysis

The most important characteristic of a trapezium that we recognize is, two sides of it AD and BC parallel to each other. This results in the two triangles $\triangle AOD$ and $BOC$ as similar because, at the diagonal intersecting point $O$ opposite angle are equal as well as opposite internal angles of intersection of AC and BD with parallel lines AD and BC are equal.

This results in equality of ratios of corresponding sides of the two triangles. We are particularly interested in the ratios involving the four diagonal sections as,

$\displaystyle\frac{3x-19}{x-5}=\frac{x-3}{3}$.

To simplify the numerator of the LHS we multiply both denominators by 3 and subtract 1 from both sides,

$\displaystyle\frac{3x-19}{3x-15}-1=\displaystyle\frac{x-3}{9}-1$,

Or, $\displaystyle\frac{-4}{x-5}=\frac{x-12}{3}$,

Or, $-12=x^2-17x+60$,

Or, $x^2-17x+72=0$,

Or, $(x-8)(x-9)=0$.

So the possible values of $x$ are 8 and 9.

Answer: b: 8, 9.

Key concepts used: Trapezium concepts -- similar triangle properties -- basic algebra concepts -- roots of a quadratic equation -- factorization of a quadratic equation.

Problem 7.

In figure below $\angle AEB=35^0$ and $\angle ADF=75^0$.

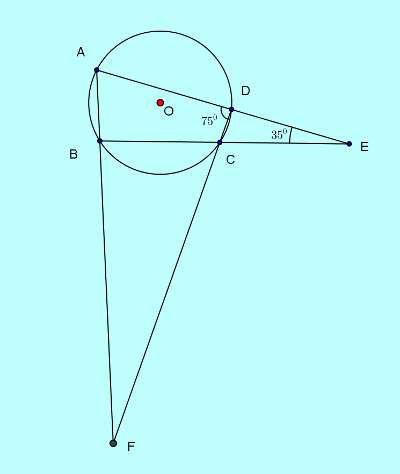
The $\angle AFD$ is,

1. $65^0$
2. $45^0$
3. $35^0$
4. $55^0$

Solution 7 - Problem analysis and solution

In $\triangle CDE$, $\angle CDE=180^0-75^0=105^0$, being an angle at the point of incidence of FD on AE forming two angles with $\angle CDA=75^0$.

So,

$\angle DCE=\angle BCF=180^0-(105^0+35^0)=40^0$, $\angle BCF$ being the opposite angle to $\angle DCE$ at the point of intersection of two lines.

Now in cyclic quadrilateral ABCD as sum of two opposite angles is $180^0$,

$\angle ABC = 180^0-75^0=105^0$.

Lastly, $\angle ABC=105^0$ is the external angle to the $\triangle BFC$, and so it equals sum of two opposite internal angles,

$\angle ABC=105^0=\angle BFC + \angle BCF$,

Or, $\angle AFD=\angle BFC=105^0-40^0=65^0$.

Answer: a: $65^0$.

Key concepts used: External angle of a triangle -- angles at point of incidence of a line -- angles at intersection of two lines -- angles in a triangle -- cyclic quadrilateral properties.

Problem 8.

In the figure below, $O$ is the centre of a circle with $\angle COD=106^0$ and AC as the diameter.

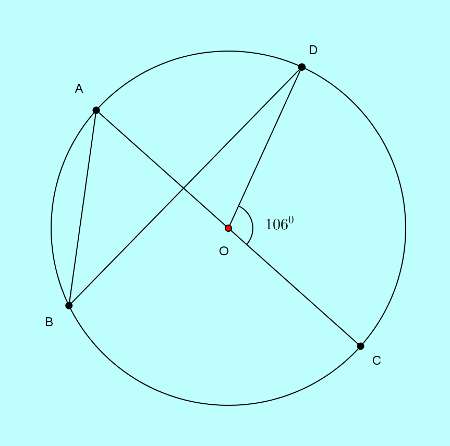
Find $\angle ABD$.

1. $55^0$
2. $40^0$
3. $37^0$
4. $43^0$

Solution 8 - Problem analysis and solving

The following figure will aid solution of the problem.

We introduce a new geometric element, that is, line BC, as a key to the solution.

By arc angle subtending concept $\angle CBD$ will be half of $\angle COD=106^0$, that is, $\angle CBD=\frac{1}{2}\text{ of  }(106^0)=53^0$.

As AC is the diameter, $\angle ABC=90^0$.

So,

$\angle ABD=90^0-53^0=37^0$.

Answer: c: $37^0$.

Key concepts used: Geometric element introduction technique -- arc angle subtending concept -- diameter angle subtending concept.

Problem 9.

In the following figure, two circles with centres at P and Q and each of radius 1 cm touch each other at O which is the centre of a third circle of radius 2 cm. The fourth circle with centre at R touches all the three circles.

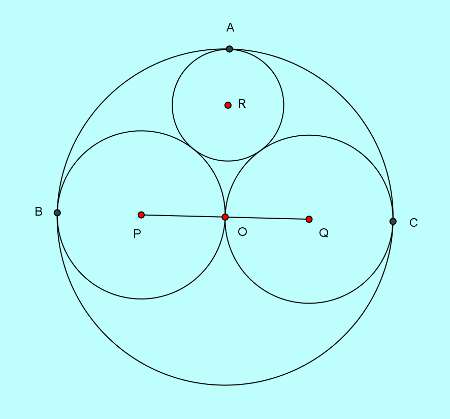
The radius of the smallest fourth circle (in cm) is,

1. $5$
2. $\displaystyle\frac{2}{3}$
3. $\displaystyle\frac{3}{5}$
4. $\displaystyle\frac{3}{7}$

Solution 9 - Problem analysis and solution

The following figure will aid the problem solution.

Let the radius of the smallest fourth circle be $r$.

As PR and QR both connect the centre of 1 cm radius circle to centre of the smallest circle passing through the common tangent points, $PR=QR=1+r$, and angle bisector of $\angle PRQ$ which is PO is perpendicular to PQ and also bisects it.

Additionally, O being the tangent point of the two 1 cm radius circles, as well as the centre of the larger 2 cm radius circle, OR extended to tangent point A, that is, OA is the radius of the larger circle.

With this background in place let us relate the sides of the $\triangle PRO$ applying Pythagoras theorem.

In $\triangle PRO$,

$PR^2=OP^2+OR^2$,

Or, $(1+r)^2=1^2+(OA-AR)^2$,

Or, $1+2r+r^2=1+(2^2-4r+r^2)$,

Or, $6r=4$,

Or, $r=\displaystyle\frac{2}{3}$ cm.

Answer: b: $\displaystyle\frac{2}{3}$.

Key concepts used: Visualization -- tangent concepts -- isosceles triangle concepts -- basic algebra concepts -- internally touching circles concept -- Pythagoras theorem -- Algebraic simplification.

Problem 10.

In a $\triangle ABC$, $AB=3$ cm, $BC=6$ cm and $CA=5$ cm. Bisector of $\angle A$ meets BC at D. The length of BD is,

1. 2.25 cm
2. 2 cm
3. 2.5 cm
4. 3 cm

Solution 10 - Problem analysis

The following is the visualization relevant to the problem.

The key pattern we identify at first is,

$BC^2=6^2=36=9+25=3^2+5^2=AB^2+AC^2$.

It means $\angle A=90^0$.

In spite of this breakthrough at the start we don't find any obvious way to relate the side lengths with each other for determining the value of BD. Under these circumstances we decided that only option is to take recourse to laws of sines finding and equating value of AD from $\triangle ABD$ and $\triangle ACD$.

Solution 10 - Problem solving execution

By laws of sines, in $\triangle ABD$,

$\displaystyle\frac{BD}{\ sin \theta}=\frac{AD}{\ sin \ B}$,

Or, $AD=\displaystyle\frac{\ sin \ B}{\ sin \theta}BD$.

Similarly applying laws of sines in $\triangle ACD$ we get,

$AD=\displaystyle\frac{\ sin \ C}{\ sin \theta}CD$.

Equating the two relations and eliminating common denominator of $\ sin \theta$ we get,

$BD\times{\ sin \ B}=CD\times{\ sin \ C}$,

Or, $\displaystyle\frac{CD}{BD}=\frac{\ sin \ B}{\ sin \ C}$.

We have used the common element AD in the two triangles to link these two parts of $\triangle ABC$. This is application of link element use technique.

Now is the time to use the basic trigonometric concept of sine-cosine complementary function relation,

$\ sin (90^0 - \theta)=\ cos \theta$.

In our case, as $\angle B+\angle C=90^0$,

$\ sin \ C=\ sin (90^0-B)=\ cos \ B$.

So,

$\displaystyle\frac{CD}{BD}=\ tan \ B=\frac{AC}{AB}=\frac{5}{3}$, as $\angle A=90^0$, our very first discovery.

Adding 1 to both sides of the equation we get,

$\displaystyle\frac{BC}{BD}=\frac{8}{3}$,

Or, $BD=\displaystyle\frac{3}{8}\times{6}=\frac{9}{4}=2.25$ cm.

Answer: a: 2.25 cm.

Key concepts used: Key pattern identification -- Pythagoras theorem -- deductive reasoning -- laws of sines -- link element use of AD to link two parts of $\triangle ABC$ -- link element use technique -- basic trigonometry concepts -- complementary trigonometric functions -- basic ratio concepts.

Note: Though this problem looks simple, it needs application of a number of rich concepts and a crucial information discovery and use. Additionally it needs trigonometry concepts.

---

Method for taking the test and get the best results from the test set:

1. Before start, go through the tutorials on Geometry basic concepts part 1 on points lines triangles, Geometry basic concepts part 2 on Quadrilaterals Squares Rectangles, Geometry basic and rich concepts part 3 on Circles, Basic and rich Geometry concepts part 4 on proof of arc angle subtending concept, Basic and rich geometry concepts part 5 on proof of median relations, Basic and rich Geometry concepts Part 6 on proof of triangle area from medians, Basic and Rich Geometry concepts part 7 on laws of sines and laws of cosines or any other short but good material to refresh your concepts if you so require.
2. Answer the questions in an undisturbed environment with no interruption, full concentration and alarm set at 15 minutes.
3. When the time limit of 15 minutes is over, mark up to which you have answered, but go on to complete the set.
4. At the end, refer to the answers given at the end to mark your score at 15 minutes. For every correct answer add 1 and for every incorrect answer deduct 0.25 (or whatever is the scoring pattern in the coming test). Write your score on top of the answer sheet with date and time.
5. Identify and analyze the problems that you couldn't do to learn how to solve those problems.
6. Identify and analyze the problems that you solved incorrectly. Identify the reasons behind the errors. If it is because of your shortcoming in topic knowledge improve it by referring to only that part of concept from the best source you get hold of. You might google it. If it is because of your method of answering, analyze and improve those aspects specifically.
7. Identify and analyze the problems that posed difficulties for you and delayed you. Analyze and learn how to solve the problems using basic concepts and relevant problem solving strategies and techniques.
8. Give a gap before you take a 10 problem practice test again.

Important: both practice tests and mock tests must be timed, analyzed, improving actions taken and then repeated. With intelligent method, it is possible to reach highest excellence level in performance.

---

Guided help on Geometry in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on Geometry in Suresolv, follow the guide,

Suresolv Geometry Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II and Other Competitive exams.

The guide list of articles includes ALL articles on Geometry and relevant topics in Suresolv and is up-to-date.

---