
Learn concepts and techniques for solving percentage basic questions quickly
How to Solve Percentage Basic Questions SSC CGL Set 69 explains concepts and techniques to solve 10 percentage practice questions easily in 12 mins time.
If you have not taken the test yet, take it first at,
SSC CGL Percentage Practice Questions Set 69.
How to solve percentage basic questions SSC CGL Set 69 - time to solve was 12 mins
Problem 1.
Instead of giving a discount, for every 19 kites sold, a kite-seller gives 1 kite extra free. In order to give 5% more discount, the number of extra kites the kite-seller needs to give free in a sale of 27 kites is,
- 3
- 6
- 8
- 7
Solution 1: Problem analysis
First task on our hands is to find the percentage discount equivalent to giving 1 kite extra free with every 19 kites sold.
Solution 1: Percentage discount equivalent to extra items given free in a sale
When $x$ number of additional items is given free in a sale of $y$ number of items, the action is equivalent to giving a discount.
In such a sale, what actually happens is,
$\text{Total marked price}=(x+y)c$, where $c$ is marked price (intended sale price) per item. Discount is given on this price.
But as $x$ items are given free,
$\text{Total sale price}=yc$.
So discount given is,
$\text{Actual discount}=(x+y)c-yc=xc$.
And the percentage discount is,
$\text{Percentage discount}=\displaystyle\frac{x}{x+y}\times{100}$.
A faster way to arrive at this percentage discount is to proceed conceptually.
$x$ out of $(x+y)$ items are given free. So,
$\text{Percentage discount}=\displaystyle\frac{x}{x+y}\times{100}$.
In our problem case, 1 kite out of $(19+1)=20$ kites is given free. So discount is simply one-twentieth or 5%.
And the target discount is 5% more, that is, 10%.
Solution 1: Problem solving
In a sale of 27 kites, if 3 kites are given free it is equivalent to giving 3 kites free out of total 30 kites given away in the sale, and that is equivalent to a discount of 10%.
It is trivial to deduce it mathematically also.
Answer: Option a: 3.
Key concepts used: Basic percentage concepts -- Discount equivalence of free items -- Mathematical reasoning -- Percentage reference concept, percentage of free items with reference to total number of free items is mapped to percentage of discount value with reference to total marked value.
Problem 2.
Two fixed points A and B are 15 cm apart. A third point C is located between the two points on the straight line joining them so that length of AC is 9 cm. If the point C is moved towards point B so that the length of AC is increased by 6%, the length of BC must have been decreased by,
- 6%
- 8%
- 7%
- 9%
Solution 2 : Problem analysis and execution
As length of AC is 9 cm out of a total of 15 cm, it is effectively,
$\displaystyle\frac{9}{15}\times{100}=60$% of the total length of AB and so BC is 40% of AB.
6% increase of length of AC is then on 60% of length of AB, which is, 3.6% of AB.
BC must have been reduced by this percentage amount. In other words, BC is reduced by 3.6% of AB while its own length is 40% of AB. Thus percentage of its own length by which BC is reduced is,
$\displaystyle\frac{3.6}{40}\times{100}=9$%.
We have equalized the base of both percentages of reduction of BC and its own length to percentages of AB so that the ratio of the two simply cancels out the length of AB and gives us percentage of reduction of length in terms of its own length.
Convention:
When it is stated that, "$x$ amount is reduced by $y$%, the percentage is to be operated on $x$.
In other words, the reference base of a percentage is always the original amount, unless otherwise mentioned.
Note: In this problem case, we have used the percentage lengths as the reference base instead of actual lengths. This has been possible because of using the percentages on the common base of total length.
Answer: Option d : 9%.
Key concepts used: Basic percentage concepts -- Base equalization technique -- Efficient simplification by operating mostly on percentages avoiding calculation of actual amounts -- Percentage as actual values -- Percentage reference concept, percentages converted to the reference base of fixed AB, and then reference is changed to percentage value of BC.
Problem 3.
In a three hour test, half way through, out of a total number of 200 questions Abhi answered 40% of the questions. What should be the percentage increase in average answer rate during the rest of the time so that Abhi can answer all the questions?
- $60$%
- $50$%
- $40$%
- $33\frac{1}{3}$%
Solution 3 : Problem analysis and execution
As the duration of the two phases of answering questions are equal, the average answer rate of the two phases are proportional to the number of questions answered in each phase.
Again, as number of questions answered is proportional to percentage of total questions answered, average answer rate is proportional to percentage of total questions answered in each phase.
So the first phase answer percentage being 40%, to answer all the questions in the second phase, Abhi needs to answer 60% of the total questions in the second phase.
In other words, the average answer rate should be 60% in the second phase, an increase of 50% of 40% in the first phase.
Answer: Option b: $50$%.
Key concepts used: Basic percentage concepts -- Basic proportionality concepts -- Basic ratio concepts -- Percentage as actual values -- Percentage change -- Mathematical reasoning.
Problem 4.
In a final exam, Vikas scores 30% but fails to pass by 5 marks, while Chandra who got 10 more marks than the pass marks scores 40%. The pass marks is then,
- 100
- 70
- 50
- 150
Solution 4 : Problem analysis and execution
Assuming pass marks to be $P$ and total marks as $T$, for Vikas,
$0.3T+5=P$.
And for Chandra,
$0.4T-10=P$.
Subtracting the first from the second,
$0.1T=15$,
Or, $T=150$.
Using this value in the first equation,
$0.3T+5=P$,
Or, $P=45+5=50$.
Answer: Option c: 50.
Key concepts used: Basic percentage concepts -- Percentage to decimal conversion -- Problem modelling -- Solving of linear equations.
Problem 5.
The price of an item was increased by 10%. This caused a reduction in monthly total item sales by 20%. The overall effect of price increase on the monthly total sale value is then,
- 10% increase
- 12% decrease
- 12% increase
- 10% decrease
Solution 5 : Problem analysis and execution
If $N_1$ and $P_1$ are the total items sold and price per item before price increase, total sale value before price increase is,
$S_1=N_1\times{P_1}$.
Similarly, if $N_2$ and $P_2$ are the total items sold and price per item after price increase, total sale value after price increase is,
$S_2=N_2\times{P_2}$.
By given conditions,
$1.1P_1=P_2$, and
$0.8N_1=N_2$.
The first and second sale value expressions are then,
$S_1=N_1\times{P_1}$, and
$S_2=N_2\times{P_2}=0.88N_1\times{P_1}$.
Taking the ratio,
$S_2 : S_1=0.88 : 1$.
Effectively then, total sale value after price increase has decreased by 12% from the original total sale value. The 20% drop in total item sales is compensated partially by the per item price increase.
Answer: Option b: 12% decrease.
Elegant solution 5: Use of the concept of Percentage change in product relation
Total sale value is equal to the product of price per item and number of items sold.
As price is increased by 10% to 1.1 times of initial price, and number of items sold reduced by 20% to 0.8 times of initial number of items sold, the total sale value after price rise effectively becomes $1.1\times{0.8}=0.88$ times initial total sale value.
This is equivalent to a 12% decrease in total sale value.
In this conceptual solution we have first used the concept of Transformation to percentage reference to express the changed values in terms of initial values and then formed the product of the decimal change coefficients of the two changed values to get the decimal change coefficient of changed product value.
This is use of the concept of Percentage change in product relation.
The same concept will be applicable for product or division of any number of factors.
For example, if a quantity is a product of two factors A and B with a third factor C dividing, when A is increased by 20%, B is decreased by 25% and C is increased by 50%, the change coefficients of the three will be, 1.2, 0.75 and 1.5 respectively. The change coefficient after the change would then be,
$\displaystyle\frac{1.2\times{0.75}}{1.5}=0.6$.
This is equivalent to a 40% reduction in the quantity after change. This is a good example of how use of a rich concept can help to solve a complex problem very easily.
Transforming the decimal change coefficient after change to percentage and getting the change percentage from 100% gives us the solution.
Key concepts used: Problem modelling to form the two total sale value expressions -- Percentage to decimal conversion -- Transformation to percentage reference concept -- Decimal change coefficient -- Percentage change in product relation -- Decimal to percentage conversion -- Basic percentage concepts.
Problem 6.
A number is increased by 20% and then again by 20%. By what percent should the inreased number be reduced to get back to the original number?
- $30\displaystyle\frac{5}{9}$%
- $44$%
- $19\displaystyle\frac{11}{31}$%
- $40$%
Solution 6 : Problem analysis and execution
Two increments by same rate of 20% is equivalent to compound growth for two periods by 20% which results in a final value after increments as,
$N_F=N(1+0.2)^2=1.44N$, where $N$ and $N_F$ are the original and final values.
To get back to the value of $N$, the reduction percentage of $N_F$ would then be,
$\displaystyle\frac{0.44N}{1.44N}\times{100}=\frac{1100}{36}=\frac{275}{9}=30\displaystyle\frac{5}{9}$%.
Answer: Option a : $30\displaystyle\frac{5}{9}$%.
Key concepts used: Compound growth concept -- Basic percentage concepts -- domain mapping.
Problem 7.
In a basic science library 25% of the books are on Bioscience, 200 are on Physics and the rest are on Chemistry. If the ratio of number of books on Chemistry, Physics and Bioscience is, 5 : 4 : 3, how many new books only on Bioscience are to be added to the library collection to make the percentage of Bioscience books as 40%?
- 75
- 150
- 100
- 125
Solution 7 : Problem analysis and execution
Using basic ratio concepts and Cancelled out HCF reintroduction technique, the actual number of Chemistry, Physics and Bioscience books may be assumed as, $5x$, $4x$ and $3x$ respectively with $x$ as the cancelled out HCF.
As number of Physics books is 200, we get the value of $x$ easily as 50.
So total number of books is, $12x=600$, and number of Bioscience books, $3x=150$.
Assuming number of Bioscience books to be purchased as $y$, by target condition,
$0.4(600+y)=150+y$,
Or, $0.6y=90$,
Or, $y=150$.
Answer: Option b: 150.
Key concepts used: Basic ratio concepts -- Cancelled out HCF reintroduction technique -- Transformation to percentage reference concept -- Basic percentage concepts -- Percentage to decimal conversion.
Problem 8.
The sum of two numbers is 520. If the larger number is decreased by 4% and the smaller number is increased by 12%, the numbers thus obtained become equal. The smaller of the two original numbers is then,
- 300
- 240
- 280
- 210
Solution 8 : Problem analysis and execution
Assuming the smaller number as $x$, by the given conditions we have,
$1.12x=0.96(520-x)$,
Or, $2.08x=0.96\times{520}$,
Or, $x=\displaystyle\frac{0.96\times{520}}{2.08}=\displaystyle\frac{96\times{40}}{16}=240$, first cancelling factor 13 and then factor 16.
Answer: Option b: 240.
Key concepts used: Problem modelling to form the crucial relationship in a single variable which has been chosen as the target variable -- Percentage to decimal conversion --Transformation to percentage reference concept -- Basic percentage concepts -- Efficient simplification -- Delayed evaluation.
Problem 9.
In an examination 65% of the students passed in Mathematics, 48% passed in Physics and 30% passed in both. How much percentage of students then failed in both subjects?
- 13%
- 17%
- 43%
- 47%
Solution 9 : Problem analysis and execution
By basic set union concept, if we add percentage of students passed in Mathematics and percentage of students passed in Physics, it will be a Union of two subsets of students passing in two subjects individually minus students who passed in both subjects. This is because just the addition of two subset values will include the percentage of students who passed in both subjects twice.
This can be shown pictorially in the Venn diagram as below,
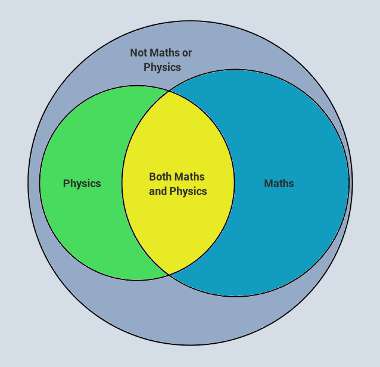
The overlapping portion of two subsets corresponding to students passed in Maths and Physics represents percentage of students who passed in both subjects.
So to get percentage of students who passed in either Maths or Physics we need to subtract the overlapping portion once (it is included twice) from the total of two subsets. Thus percentage of students who passed either in Maths or Physics is,
$65\text{%}+48\text{%}-30\text{%}=83\text{%}$.
The percentage of students who failed in both Maths and Physics is then the rest of the students who didn't pass either in Maths or in Physics, that is,
$100\text{%}-83\text{%}=17\text{%}$.
This last operation is a set exclusion operation that is equivalent to arithmetic subtraction. The "either-or Maths Physics subset of 83%" is subtracted from the whole set of 100% to get the "not any of Maths Physics subset" of 17%.
Total number of students is represented by 100% and all other number of students are in terms of this 100%. Thus instead of actual number of students we could deal with percentage of students for all the subsets.
Answer: Option b: 17%.
Key concepts used: Basic set union concept -- Venn diagram -- Basic percentage concepts -- Basic set theoretic concepts -- Percentage as actual values.
Problem 10.
A's salary is 40% of B's salary and B's salary is 25% of C's salary. What percent of C's salary is A's salary?
- 15%
- 5%
- 20%
- 10%
Solution 10 : Problem analysis and execution
Assuming C's salary as 100, B's salary is 25% of 100, that is 25.
A's salary is 40% of B's salary, that is, 40% of 25 which is 10.
So A's salary is 10% of C's salary.
Answer: Option d: 10%.
Key concepts used: Basic percentage concepts -- Percentage of percentage concept -- Working backwards approach -- Mathematical reasoning.
Resources that should be useful for you
7 steps for sure success in SSC CGL tier 1 and tier 2 competitive tests or section on SSC CGL to access all the valuable student resources that we have created specifically for SSC CGL, but generally for any hard MCQ test.
Concept Tutorials on related topics
Basic concepts on fractions and decimals part 1
Basic concepts on Ratio and Proportion
Componendo dividendo applied on number system and ratio proportion problems
How to solve problems on mixing liquids and based on ages
Basic and Rich Percentage Concepts for solving difficult SSC CGL problems
Efficient solution techniques on related topics
How to solve SSC CGL level arithmetic mixture problems in a few simple steps 1
How to solve SSC CGL level arithmetic mixture problems in a few simple steps 2
How to solve SSC CGL level number and age ratio problems lightning fast
How to solve a tricky SSC CGL level age ratio problem lightning fast
SSC CGL level solved question sets on mixture or alligation
SSC CGL level solved questions sets 78 on mixture or alligation 1
SSC CGL level solved question set 85 on mixture or alligation 2
SSC CHSL level solved question sets on Mixture or Alligation
SSC CHSL level Solved Question set 9 on Mixture or Alligation 1
SSC CHSL level Solved Question set 10 on Mixture or Alligation 2
SSC CGL Tier II level solved question sets on mixture or alligation
SSC CGL Tier II level solved question set 24 on mixture or alligation 1
SSC CGL Tier II level solved question set 25 on mixture or alligation 2
SSC CGL Tier II level question and solution sets on Ratio and Proportion
SSC CGL Tier II level Solution Set 23 Ratio proportion 2
SSC CGL Tier II level Question Set 23 Ratio proportion 2
SSC CGL Tier II level Solution Set 22 Ratio proportion 1
SSC CGL Tier II level Question Set 22 Ratio proportion 1
Other SSC CGL question and solution sets on Ratio and Proportion and Percentage
SSC CGL level Solution Set 84, Ratio proportion 8
SSC CGL level Question Set 84, Ratio proportion 8
SSC CGL level Solution Set 83, Ratio Proportion 7
SSC CGL level Question Set 83, Ratio Proportion 7
SSC CGL level Solution Set 76, Percentage 4
SSC CGL level Question Set 76, Percentage 4
SSC CGL level Solution Set 69, Percentage 3
SSC CGL level Question Set 69, Percentage 3
SSC CGL level Solution Set 68, Ratio Proportion 6
SSC CGL level Question Set 68, Ratio Proportion 6
SSC CGL level Solution Set 31, Ratio and Proportion 5
SSC CGL level Question Set 31, Ratio and Proportion 5
SSC CGL Level Solution Set 25, Percentage, Ratio Proportion 4
SSC CGL level Question Set 25, Percentage, Ratio Proportion 4
SSC CGL level Solution Set 24 Arithmetic Ratio Proportion 3
SSC CGL level Question Set 24, Arithmetic Ratio Proportion 3
SSC CGL level Solution Set 5 Arithmetic Ratio Proportion 2
SSC CGL level Question Set 5 Arithmetic Ratio Proportion 2
SSC CGL level Solution Set 4 Arithmetic Ratio Proportion 1
SSC CGL level Question Set 4, Arithmetic Ratio Proportion 1.